| |
For real numbers  , , 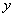 and and 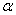 > 0 , the inequality | > 0 , the inequality |  - -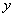 | | 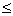 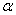 means that means that
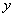 - - 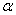 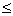  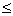 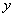 + + 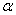 , ,
i.e.,  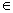 [ [ 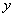 - - 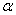 , , 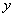 + + 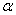 ] . ] . |
1.2.8 |
Definitions (Algebra of real valued functions) : |
|
|
|
|
|
(v) For any integer 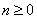 and real numbers and real numbers , the function , the function 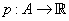 defined by defined by
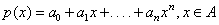 , ,
is called a polynomial function. When every 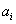 is an integer, it is called an integral polynomial function. is an integer, it is called an integral polynomial function. |