(vi) |
A function 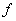 is called bijective if it is injective as well as surjective. is called bijective if it is injective as well as surjective. |
(vii)
|
If 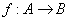 and and 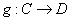 with with 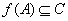 , then the composite function is the function go , then the composite function is the function go 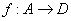 defined by defined by 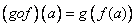 for for 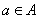 . . |
| |
For 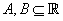 , saying that a function , saying that a function 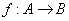 , is onto means that the horizontal line at every point , is onto means that the horizontal line at every point 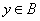 meets the graph of the function at least once. Similarly, saying that the function is one -one means that the horizontal line at every point meets the graph of the function at least once. Similarly, saying that the function is one -one means that the horizontal line at every point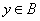 meets the graph of the function at most once. meets the graph of the function at most once. |
| |
Click here to see an interactive visualization: Applet 1.1 |
| |
Click here to see an interactive visualization: Applet 1.2 |
| |
The function 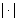 : : 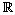 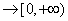 defined by defined by

is called the absolute value function and |  | is called the absolute value of | is called the absolute value of  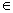 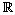 . .
|
| |
For every  the following results are true: the following results are true: |
| (i) |
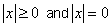 if and only if if and only if  , ( that is, , ( that is, 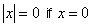 , and conversely , and conversely 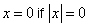 ). ). |