9 End Torsion of Prismatic Bars

Chapter 9
End Torsion of Prismatic Bars

9.1 Overview

In the last chapter, we studied the response of straight prismatic beams subjected to bending moments. In this chapter, we study the stresses that develop and the displacement that these members undergo when subjected to twisting moment. While in the analysis of beams subjected to bending moments we maintained some generality with the loading, the study of torsion is focused on a single loading case - one end fixed and the other end free to twist, as shown in figure 9.1. Here the double arrow indicates the twisting moment. However, we study this boundary value problem for a variety of cross sections. This analysis for torsion depends on whether the section is thick walled or thin walled as in the case of bending moments. It also depends on whether the section is open or closed. We shall discuss in detail as to when a section is closed subsequently.

Figure 9.1: Schematic of a straight prismatic member subjected to end torsion

Before proceeding further, we would like to point out the change in the orientation of the coordinate system, from that used in the study of the beams. This is necessitated because for some problems we would be using cylindrical polar coordinates, and want the cross section to be in the xy plane. We use cylindrical polar coordinates with this orientation so that the boundary of the cross section can be defined easily. Since, the value of displacement or stress in a material particle is independent of the coordinate system used, it is expected that the analyst pick coordinate systems that is convenient for a problem and not that which he is comfortable with. Hence, the change.

For this orientation of the coordinate system, following the same steps as discussed in detail in chapter 8, it can be shown that the torsional moment, M_z is given by,

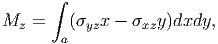∫ Mz = (σyzx - σxzy)dxdy, a

(9.1)

and for completeness, the other two components of the moment, which are bending moments are,

∫ My = - σzzxdxdy, (9.2) ∫ a M = σ ydxdy. (9.3) x a zz

Comparing equations (9.1) with (9.2) and (9.3) it can be seen that while the bending moments are due to normal stresses, torsional moment is due to shear stresses. This is the major difference between the bending and twisting moment. While the bending moment gives raise to normal stresses predominantly, twisting moment gives raise to shear stresses predominantly.

Now let us see how to classify the sections. As in case of bending, a cross section would be classified as thin walled if the thickness of the cross section is such that it is much less than the characteristic dimensions of the cross section. Typically, if the ratio of the thickness of the cross section to the length of the member is less than 0.1, the section is classified as thin. If the section is not thin, it is considered to be thick.

Figure 9.2: Section classified as closed sections when subjected to torsion

Figure 9.3: Section classified as open sections when subjected to torsion

If a section when twisted can deform such that plane sections before deformation remain plane after twisting is called closed section. Sections which do not deform in the above manner are called open sections. Solid Circular cross section is an example of closed section and solid rectangular cross section is an example of open sections. Similarly, a thin walled annular cylinder (see figure 9.2) is an example of closed section and if the same annular cylinder has a longitudinal slit (see figure 9.3), it is an example of open section. All closed section allows for continuous variation of shear stresses such that it is no where zero except at the centroid of the cross section. A section which requires the shear stresses to be zero at locations apart from the centroid of the cross section, like at the corners of a rectangular cross section, is an open section. It should be pointed out that in elliptical shaped cross section also the shear stress is zero only at the centroid of the cross section but is an open section as plane sections do not remain plane after twisting. Thus, the requirement on the shear stress is just necessary but not sufficient to classify a given section as closed.

In the following section, we find the displacement field and stress field for twisting of a thick walled sections and then focus on thin walled sections.

9.2 Twisting of thick walled closed section

First, we study the twisting of thick walled closed section and then thin walled closed section. This problem we study using the displacement approach. We also use cylindrical polar coordinates to formulate and study the problem. While the solution that we obtain does not require that the cross section to be closed section be circular, we shall assume this for illustration purpose, mainly to fix the geometry of the body. Thus, we assume the body to be an right circular annular (or solid) cylinder occupying a region in the Euclidean point space given by: = {(r,θ,z)|R_i ≤ r ≤ R_o, 0 ≤ θ ≤ 2π, 0 ≤ z ≤ L}, where R_i, R_o and L are constants with R_i = 0 in case of solid cylinder. Since, we are interested in this body being subjected to pure torsional moment, the traction boundary conditions are

t(er)(Ro, θ,z) = o, t(er)(Ri,θ, z) = o, (9.4) ∫ ∫ t(- ez)(r,θ,0)da = o, t(ez)(r,θ,L)da = o, (9.5) ∫ a ∫a a rer ∧ t(-ez)(r,θ,0)da = - Tez, a rer ∧ t(ez)(r,θ,L )da = T ez,(9.6)

where T is a constant. Further, since one end of this body is assumed to be fixed and the other end free to displace radially and circumferentially, the displacement boundary conditions for this problem is,

u(r,θ,0) = o, u (r,θ,L) = 0, z

(9.7)

where u_z denotes the z component of the displacement field and we have assumed that the surface of the body defined by z = 0 is fixed and the other surfaces are free to displace radially and circumferentially.

(a) Schematic of deformation in the yz plane 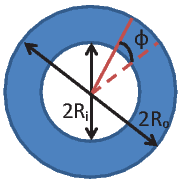(b) Schematic of deformation in the xy plane

Figure 9.4: Schematic of the deformation of a straight prismatic member subjected to end torsion

Since, we are going to solve the problem using the displacement approach, we have to assume the displacement field. We shall assume that plane sections normal to the axis of the member, e_z remain plane and perpendicular to the axis. This means that the z component of the displacement field is only a function of z, i.e., u_z = û_z(z). Further, the boundary condition (9.7) requires that u_z(0) = u_z(L) = 0. Consistent with these requirements, we assume that the z component of the displacement, u_z = 0. We could have also assumed u_z = C sin(mπz∕L), where m is an integer and C is a constant. However, it can be shown that such an assumption to satisfy balance of linear momentum would require that C = 0. Then, we shall assume that there is no radial component of the displacement, i.e., u_r = 0. Since, straight radial lines before deformation remain as straight radial lines after deformation with their length unchanged, we are justified in making the assumption that u_r = 0. Further, we assume that the straight line along the axis of the member remains straight but rotates by an angle θ and that the radial lines rotate by an angle ϕ as shown in the figure, 9.4 when subjected to twisting moment. Consequently, the circumferential component of the displacement, u_θ = Ωrz, where Ω is called as the angle of twist per unit length. The slope of the straight line along the axis of the member located at a radial distance, r^* after deformation, α = Ωr^*. Similarly, the rotation undergone by radial lines in a plane defined by z = z^*, where z^* is a constant, is ϕ = Ωz^*. Thus, the displacement field is taken as,

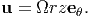u = Ωrze θ.

(9.8)

It can then be verified that this displacement field satisfies the displacement boundary conditions (9.7). Then, the strain field corresponding to the displacement field (9.8) is

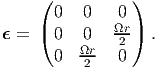( ) 0 0 Ω0r ϵ = ( 0 0 2 ) . 0 Ωr2 0

(9.9)

Using isotropic Hooke’s law, (7.2) the stress corresponding to the strain (9.9) is computed as

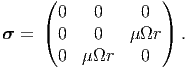( ) 0 0 0 σ = ( 0 0 μ Ωr ) . 0 μΩr 0

(9.10)

It can then be verified that the above state of stress, satisfies the static equilibrium equations in the absence of body forces, (7.6) trivially. It can also be verified that the stress given in equation (9.10) satisfies the boundary condition (9.4) trivially. For the stress state (9.10) the boundary condition (9.5) evaluates to

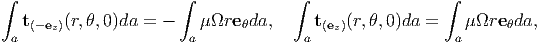∫ ∫ ∫ ∫ t(- ez)(r,θ,0)da = - μ Ωreθda, t(ez)(r,θ,0 )da = μ Ωre θda, a a a a

(9.11)

Since, the direction of e_θ changes with the location as shown in figure 9.5, we write it in terms of the fixed Cartesian basis and obtain

∫ Ro ∫ 2π ± μΩr2dr [- sin(θ)ex + cos(θ)ey]dθ = o. Ri 0

(9.12)

Thus, the boundary condition (9.5) holds for the stress state (9.10).

Figure 9.5: Schematic of variation of e_θ over the circumference of the cylinder.

Then, using the boundary condition (9.6) we obtain the relationship between the applied torque, T and the angle of twist per unit length, Ω as,

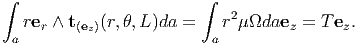∫ ∫ rer ∧ t(e)(r,θ,L )da = r2μ Ωdaez = Tez. a z a

(9.13)

Thus,

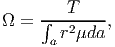T Ω = ∫-------, ar2μda

(9.14)

which for homogeneous sections reduces to

T μ Ω = --, J

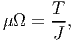(9.15)

where

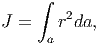∫ 2 J = r da, a

(9.16)

is the polar moment of inertia. Combining equations (9.10) and (9.15) we obtain

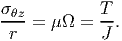σθz T --- = μΩ = --. r J

(9.17)

This is called the torsion equation.

9.2.1 Circular bar

Figure 9.6: Solid circular cross section of a bar

As discussed, for illustration, we consider a bar with solid circular cross section of radius R, as shown in figure 9.6. From the general expression for torsion of a thick walled close section (9.17), we find that the shear stresses in the bar is given by,

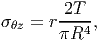-2T- σθz = rπR4 ,

(9.18)

where we have computed J = ∫ ₀^2πdθ ∫₀^Rr³dr. Thus, we find that the shear stress varies linearly with the radial distance, as shown in figure 9.7.

Figure 9.7: Shear stresses in a bar with solid circular cross section subjected to end torsion

Then, the angle of twist per unit length, Ω is also obtained from (9.17) as,

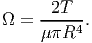Ω = --2T--. μ πR4

(9.19)

Having determined the angle of twist per unit length, the entire displacement field corresponding to an applied torque, T given by equation (9.8) is known. The same is plotted in figure 9.8.

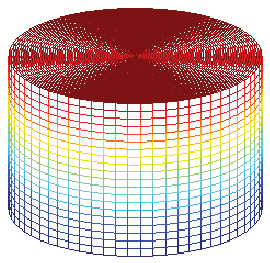(a) Undeformed circular bar 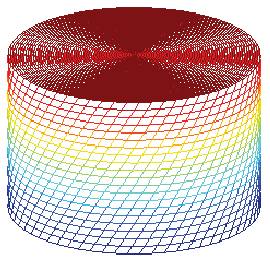(b) Deformed circular bar

Figure 9.8: Deformation of a bar with circular cross section subjected to end torsion

9.3 Twisting of solid open section

In this section, as in the previous, we consider a bar subjected to twisting moments at its ends. The bar axis is straight and the shape of the cross section is constant along the axis. The bar is assumed to have a solid cross section, i.e, a simply connected domain. A domain is said to be simply connected if any closed curve in the domain can be shrunk to a point in the domain itself, without leaving the domain. A domain which is not simply connected is said to be multiply connected. We shall analyze multiply connected domains in the next section.

Though the problem formulation does not assume any specific shape for the cross section, the displacement and stress field obtained are cross section specific. Let us assume that the boundary of the cross section is defined by a function, f(x,y) = 0. This function for a ellipse centered about the origin and major and minor axis oriented about the e_x and e_y directions would be, f(x,y) = x²∕a² + y²∕b² - 1. Consequently, for the body is assumed to occupy a region in Euclidean point space, defined by = {(x,y,z)|f(x,y) ≤ 0, 0 ≤ Z ≤ L}, where L is a constant. Here we are assuming that the bar is a solid cross section with the cross section having only one surface, f(x,y) = 0 denoted by ∂. To be more precise, the cross section is simply connected. Since, we are interested in the case where in the bar is subjected to pure end torsional moment, the traction boundary conditions are:

∫ t(en)(∂A, z ) = o, (9.20) at(-ez)(x,y,0)da = o, (9.21) ∫ t(ez)(x,y, L)da = o, (9.22) ∫ a (xex + yey) ∧ t(-ez)(z,y,0)da = - Tez, (9.23) ∫a (xex + yey) ∧ t(e )(x,y, L)da = T ez, (9.24) a z

where

∂f ∂f --[∂y-ex --∂xey]-- en = ∘ (---)---(---)2-, ∂f- 2 + ∂f ∂x ∂y

(9.25)

and T is a constant. Here e_n is obtained such that it is in the xy plane, perpendicular to grad(f) and is a unit vector. Recognize that grad(f) gives the tangent vector to the cross section for any point in ∂. The orientation of e_n and grad(f) is as shown in figure 9.9. Further, since one end of this body is assumed to be fixed against twisting but free to displace axially and the other end free to displace in all directions, the displacement boundary conditions for this problem is,

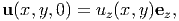u(x,y, 0) = uz(x,y)ez,

(9.26)

where u_z is any function of x and y. Here we have assumed that the surface of the body defined by z = 0 is fixed against twisting but free to displace axially and the other surfaces are free to displace in all directions.

Figure 9.9: Boundary conditions at the surface of a bar subjected to end torsion

In a solid cross section, as a result of a twist, each cross section undergoes a rotational displacement about the z axis. For any two cross sections the relative angle of rotation is called the angle of twist between the two sections. Let Δβ be the angle of twist for two cross sections at distance Δz and Ω be the angle of twist per unit length of the bar, then

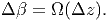Δ β = Ω(Δz ).

(9.27)

Since the conditions are same for all cross sections, the above equation applies to any two cross sections at a distance Δz along the bar length. Thus, Ω is a constant along the bar length. Also, since at z = 0, β = 0, by virtue of the surface defined by z = 0 being fixed against rotation, the angle of twist, β for a cross sections at distance z from the surface defined by z = 0 is

β = Ωz.

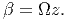(9.28)

Figure 9.10: Displacements in the cross section of a bar subjected to end twisting moment

Consider a cross section at a distance z from the fixed end. In that section consider a point A with coordinates (x,y,z). Assuming the origin of the coordinate system to be located at the axis of twisting, let r denote the radial distance of the point A from the origin and let θ denote the angle that this radial line makes with the e_x, as shown in the figure 9.10. Let A move to A′ due to twisting of the bar, as shown in the figure 9.10. The arc length AA′ would be equal to rβ, where β is the angle of twist at the cross section and is related to the angle of twist per unit length through (9.28). Hence, the arc length AA′ would be,

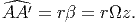^ ′ AA = rβ = rΩz.

(9.29)

Since, the angle of twist β is small, we approximate the secant length, i.e., the length of the straight line between AA′ with its arc length. Consequently, the x component of the displacement of A is given by,

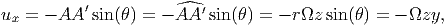ux = - AA ′sin(θ) = - ^AA ′sin(θ) = - r Ωz sin(θ) = - Ωzy,

(9.30)

where we have used (9.29) and the relation that y = r sin(θ). Similarly, the y component of the displacement of A is,

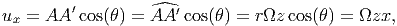ux = AA ′cos(θ) = ^AA ′cos(θ) = rΩz cos(θ) = Ωzx,

(9.31)

where as before we used (9.29) and the relation x = r cos(θ). Since, there is no restraint against displacement along e_z direction at any section, all cross sections is assumed to undergo the same displacement along the z direction and hence

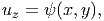uz = ψ(x,y ),

(9.32)

where ψ is a function of x and y only. That is, we have assumed that the z component of the displacement is independent of the axial location of the section. Presence of this z component of the displacement is the characteristic of open sections. It is due to this z component of the displacement the plane section distorts and is said to warp. Consequently, ψ is called Saint-Venant’s warping function. Thus, the displacement field for a bar subjected to twisting moments at the end and is free to warp is given by,

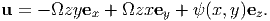u = - Ωzye + Ωzxe + ψ (x,y)e . x y z

(9.33)

It can be easily verified that the above displacement field (9.33) satisfies the displacement boundary condition (9.26).

Substituting equation (9.33) in the strain-displacement equation (7.1), the Cartesian components of the strain are computed to be

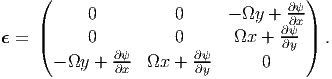( ) 0 0 - Ωy + ∂∂ψx- ϵ = |( 0 0 Ωx + ∂ψ- |) . ∂ψ- ∂ψ-- ∂y - Ωy + ∂x Ωx + ∂y 0

(9.34)

For this state of strain, the corresponding Cartesian components of the stress are obtained from Hooke’s law (7.2) as

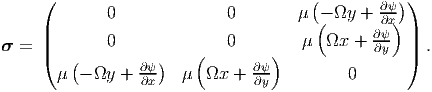( ( )) 0 0 μ - Ωy + ∂ψ- | ( ∂∂ψx) | σ = |( 0 ( 0 ) μ Ωx + ∂y |) . ( ∂ψ-) ∂ψ- μ - Ωy + ∂x μ Ωx + ∂y 0

(9.35)

For this stress state, (9.35) to be possible in a body in static equilibrium, without any body force acting on it, it should satisfy the balance of linear momentum equations (7.6) which requires that,

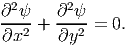2 2 ∂-ψ- + ∂-ψ- = 0. ∂x2 ∂y2

(9.36)

Now, we have to find ψ such that equation (9.36) holds along with the prescribed traction boundary conditions (9.20) through (9.24).

Boundary condition (9.20) requires

[ ] [ ] μ - Ωy + ∂ψ- ∂f-- μ Ωx + ∂-ψ ∂f- = 0, ∂x ∂y ∂y ∂x

(9.37)

for (x,y) ∈ ∂. Both the boundary conditions (9.21) and (9.22) yield the following restriction on ψ,

∫ [ ] ∫ [ ] ∂ψ- ∂-ψ μ - Ωy + ∂x da = 0, μ Ωx + ∂y da = 0, a a

(9.38)

On assuming that the bar is homogeneous and appealing to Green’s theorem (section 2.9.3), equation (9.38) reduces to,

∫ [ ∂ψ ] ∮ ∂f μ - Ωy + --- da = - μΩycA + ψ∘-------∂y-------ds = 0, (9.39) a ∂x c (∂f)2 (∂f)2 ∂x + ∂y ∫ [ ] ∮ ∂f μ Ωx + ∂ψ- da = μΩx A - ψ∘-------∂x-------ds = 0, (9.40) a ∂y c c ( )2 ( )2 ∂∂fx + ∂∂fy

where (x_c,y_c) is the coordinates of the centroid of the cross section. On assuming that the axis of twisting coincides with the centroid and further requiring that the origin of the coordinate system coincide with the centroid of the cross section, equations (9.39) and (9.40) requires,

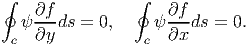∮ ∮ ψ ∂f-ds = 0, ψ ∂fds = 0. c ∂y c ∂x

(9.41)

Finally, the boundary conditions (9.23) and (9.24) require that

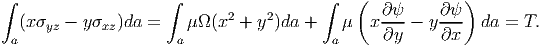∫ ∫ ∫ ( ) 2 2 ∂ψ ∂ψ (x σyz - y σxz)da = μΩ (x + y )da + μ x ---- y--- da = T. a a a ∂y ∂x

(9.42)

Recognizing that ∫ _a(x² + y²)da = J, polar moment of inertia equation (9.42) can be simplified as,

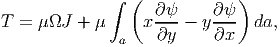∫ ( ∂ψ ∂ψ ) T = μΩJ + μ x ---- y--- da, a ∂y ∂x

(9.43)

where we have used the assumption already made that the bar is homogeneous. For many cross sections, ∫ _a ( )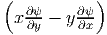
x∂∂ψy-- y ∂∂ψx- da < 0. Thus, the torsional stiffness¹ which would be μJ, in the absence of warping (i.e., ψ = 0), decreases due to warping (i.e., when ψ ≠ 0). Hence, warping is said to decrease the torsional stiffness of the cross section. Equation (9.43) is used find Ω.

Experience has shown that it is difficult to find ψ that satisfies the governing equation (9.36) along with the boundary conditions (9.37) and (9.41). Hence, we recast the problem using Prandtl stress function.

Stress function formulation

Defining a differentiable function, ϕ = 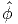(x,y) called the Prandtl stress function, we relate the components of the stress to this stress function as,

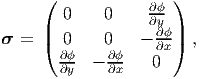( ) 0 0 ∂∂ϕy σ = |( 0 0 - ∂ϕ|) , ∂ϕ ∂ϕ ∂x ∂y - ∂x 0

(9.44)

so that the equilibrium equations (7.6) are satisfied for any choice of ϕ.

Now, one can proceed in one of two ways. Follow the standard approach and find the strain corresponding to the stress state (9.44), substitute the same in the compatibility conditions and find the governing equation that ϕ should satisfy. Here we obtain the same governing equation through an alternate approach.

Equating the stress states (9.35) and (9.44) as it represents for the same boundary value problem, we obtain

[ ] ∂ϕ ∂ψ --- = μ - Ωy + --- , (9.45) ∂y [ ∂x ] ∂ϕ- ∂ψ- ∂x = - μ Ωx + ∂y . (9.46)

Differentiating equation (9.45) with respect to y and equation (9.46) with respect to x and adding the resulting equations, we obtain

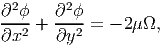2 2 ∂-ϕ-+ ∂-ϕ-= - 2μ Ω, ∂x2 ∂y2

(9.47)

where we have made use of the requirement that ∂2ψ
∂x∂y = ∂2ψ
∂y∂x . By virtue of the stress function being smooth enough so that -∂2ϕ
∂x∂y = ∂2ϕ-
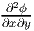∂y∂x , the governing equation for ψ (9.36) is trivially satisfied.

The boundary condition given in equation (9.37) in terms of the warping function, ψ is rewritten in terms of the Prandtl stress function, ϕ using equations (9.45) and (9.46) as,

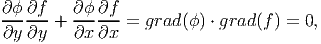∂ϕ ∂f ∂ϕ ∂f ∂y-∂y- + ∂x-∂x- = grad (ϕ ) ⋅ grad (f) = 0,

(9.48)

for (x,y) ∈ ∂. Since, grad(f) ≠ o and its direction changes with the location on the boundary of the cross section, grad(ϕ) = o on the boundary of the cross section. This implies that

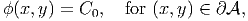ϕ(x,y) = C0, for (x,y ) ∈ ∂A,

(9.49)

where C₀ is a constant. Since, the stress and displacement fields depend only in the derivative of the stress potential and not its value at a location, it suffices to find this stress function up to a constant² . Therefore, we arbitrarily set C₀ = 0, understanding that it can take any value and that the stress and displacement field would not change because of this. Hence, equation (9.49) reduces to requiring,

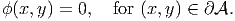ϕ (x,y) = 0, for (x,y) ∈ ∂A.

(9.50)

The boundary condition (9.21) and (9.22) for the stress state (9.44) require

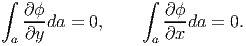∫ ∫ ∂ϕda = 0, ∂-ϕda = 0. a ∂y a∂x

(9.51)

Appealing to Green’s theorem for simply connected domains, the above equation (9.51) reduces to

∫ ∮ ∮ ∂ ϕ ∂f ∂f -∂yda = ϕ ∂x-ds = C0 ∂x-ds = 0, (9.52) ∫a ∮c ∮c ∂-ϕda = ϕ ∂f-ds = C ∂f-ds = 0, (9.53) a∂x c ∂y 0 c∂y

where we have used (9.49) and the fact that ∮ _c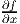 ∂f
∂x

ds = 0 and ∮ _c ∂f
∂y

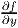ds = 0 for closed curves.

Finally, the boundary conditions (9.23) and (9.24) for the stress state (9.44) yields,

∫ [ ] - x∂-ϕ + y∂-ϕ da = T. a ∂x ∂y

(9.54)

Noting that

∫ ∫ [ ] ∮ ∫ ∫ ∂ϕ ∂(xϕ) ∂f x ---= ------- ϕ da = xϕ ---ds - ϕda = - ϕda, (9.55) ∫a ∂x ∫a[ ∂x ] ∮c ∂y ∫a ∫ a ∂ϕ- ∂(yϕ-) ∂f- a y∂y = a ∂y - ϕ da = cyϕ ∂x ds - aϕda = - aϕda, (9.56)

where we have used the Green’s theorem for simply connected domain and equation (9.50). Substituting the above equations in (9.54) we obtain,

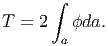∫ T = 2 ϕda. a

(9.57)

Thus, we have to find ϕ such that the governing equation (9.47) has to hold along with the boundary condition (9.50). Then, we use equation (9.57) to find the torsional moment required to realize a given angle of twist per unit length Ω.

Membrane analogy

The governing equation (9.47) along with the boundary condition (9.50) and (9.57) is identical with those governing the static deflection under uniform pressure of an elastic membrane in the same shape as that of the member subjected to torsion. This fact creates an analogy between the torsion problem and elastic membrane under uniform pressure. This analogy is exploited to get the qualitative features that the stress function should posses and this aids in developing approximate solutions. Obtaining the governing equation for the static deflection of an elastic membrane under uniform pressure and showing that it is identical to (9.47) is beyond the scope of this lecture notes. One may refer Sadd [4] for the same.

In the following three sections, we apply the above general framework to solve problems of bars with specific cross section shapes subjected to end torsion.

9.3.1 Solid elliptical section

The first cross section shape that we consider is that of an ellipse. That is we study a bar with elliptical cross section, whose boundary is defined by the function,

x2 y2 f(x,y) = -2 + -2-- 1 = 0, a b

(9.58)

where a and b are constants and we have assumed that the major and minor axis of the ellipse coincides with the coordinate basis, as shown in the figure 9.11.

Figure 9.11: Elliptical cross section of a bar

Choosing the Prandtl stress function to be,

[ ] x2 y2 ϕ = C -2-+ -2-- 1 , a b

(9.59)

where C is a constant, we find that it satisfies the boundary condition (9.50). Substituting the stress function, (9.59) in the equation (9.47) we obtain

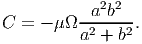a2b2 C = - μΩ -------. a2 + b2

(9.60)

Using (9.59) and (9.60) in (9.57), twisting moment is obtained as

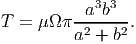a3b3 T = μ Ωπ -2----2. a + b

(9.61)

Corresponding to the stress function (9.59) the shear stresses are computed to be

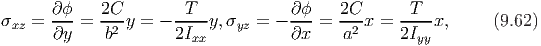
∂ ϕ 2C T ∂ϕ 2C T σxz = --- = ---y = - ----y,σyz = - --- = ---x = ----x, (9.62) ∂y b2 2Ixx ∂x a2 2Iyy

where I_xx = πab³∕4, I_yy = ba³∕4 and we have substituted for the constant, C from equation (9.60). The variation of these shear stresses over the cross section is indicated in figure 9.12.

Figure 9.12: Shear stresses in a bar with elliptical cross section subjected to end torsion

The resultant shear stress in the xy plane is given by,

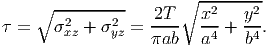∘ --------- ∘ --2----2 τ = σ2 + σ2 = 2T-- x--+ y-. xz yz πab a4 b4

(9.63)

It is clear from equation (9.63) that the extremum shear stress occurs at (0, 0) or the boundary of the cross section. It can be seen that at (0, 0) minimum shear stress occurs, as τ = 0 at this location. At the boundary the extremum shear stresses would occur at (0,±b) and (±a, 0). Thus, the extremum shear stresses are τ_ext = 2T∕(πab²) at (0,±b) and τ_ext = 2T∕(πba²) at (±a, 0). If a > b, then the maximum shear stress in the elliptical cross section is,

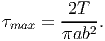-2T-- τmax = πab2 .

(9.64)

Substituting (9.59) in equations (9.45) and (9.46) and rearranging we obtain

[ ] ∂ψ- 2C-- ∂ψ- 2C-- ∂x = Ωy + μb2y, ∂y = - Ωx + μa2 x .

(9.65)

Solving the differential equations (9.65) we obtain

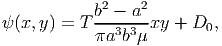2 2 ψ (x,y) = T b---a--xy + D0, πa3b3μ

(9.66)

where D₀ is a constant. Since, there is no rigid body translation, we require that ψ(0, 0) = 0. Hence, D₀ = 0. Thus, we find that the section warps into a diagonally symmetric surface as shown in figure 9.13.

Rearranging the equation (9.61) we can get Ω as a function of torque, T as

T[a2 + b2] Ω = -----3-3-- μπa b

(9.67)

The entire displacement field, (9.33) for a bar with elliptical cross section is now known that we have found the warping function (9.66). The deformation of a bar with the elliptical cross section computed using this displacement field is shown in figure 9.14.

Figure 9.13: Warping deformation of a elliptical cross section due to end torsion

(a) Undeformed elliptical bar (b) Deformed elliptical bar

Figure 9.14: A bar with elliptical cross section subjected to end twisting moment

Before concluding this section let us see the error that would have been made if one analyzes the elliptical section as a closed section. The polar moment of inertia for the elliptical section could be computed and shown to be J = πab(a² + b²)∕4. Then, the angle of twist per unit length computed from (9.17) would be,

Ω = -----4T------. cl μ πab[a2 + b2]

(9.68)

The ratio of Ω_cl∕Ω, computed from equations (9.68) and (9.67) is

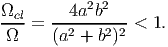Ωcl 4a2b2 --- = --2----2-2 < 1. Ω (a + b )

(9.69)

Thus, for a given torque open section twists more.

Next, we examine the shear stresses. If one uses (9.17) to compute the shear stresses, they would erroneously conclude that the maximum shear stress occurs at (±a, 0), by virtue of these points being farthest from the center of twist and the value of this maximum shear stress would be,

4T τcmlax = ----2---2-- πb[a + b ]

(9.70)

Comparing equations (9.64) and (9.70) we find that

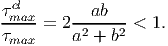τcl ab -max-= 2 -2----2 < 1. τmax a + b

(9.71)

Thus, closed section underestimates the stresses in the bar.

Hence, from both strength and serviceability point of view assuming the elliptical cross section to be closed would result in an unsafe design.

9.3.2 Solid rectangular section

Figure 9.15: Rectangular cross section of a bar

Next, we assume that the bar has a rectangular cross section of width 2b and depth 2h, as shown in figure 9.15. For this section the boundary is defined by the function,

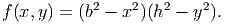f (x, y) = (b2 - x2)(h2 - y2).

(9.72)

It can be verified that choosing the Prandtl stress function, ϕ as Cf(x,y) would not satisfy the governing equation (9.47). Hence, we assume a stress function of the form,

2 2 ϕ = μ Ω[V (x,y) + b - x ],

(9.73)

where V is a function of (x,y).

Substituting equation (9.73) in equation (9.47) and simplifying we obtain,

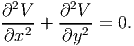∂2V ∂2V ---2-+ ---2-= 0. ∂x ∂y

(9.74)

Then, the boundary condition (9.50) requires that

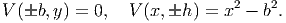V (±b, y) = 0, V (x,±h ) = x2 - b2.

(9.75)

Thus, we need to solve the differential equation (9.74) subject to the boundary condition (9.75).

Towards this, we seek solution of the form

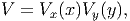V = Vx(x)Vy(y),

(9.76)

where V _x is a function of x and V _y is a function of y alone. Substituting the assumed special form for V , (9.76) in equation (9.74) and simplifying we obtain,

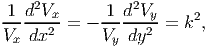1 d2Vx 1 d2Vy 2 ------2-= - -----2- = k , Vx dx Vy dy

(9.77)

where k is any constant. Recognize that the left hand side of the equation is a function of x alone and the right hand side is a function of y alone. Hence, for these functions to be same, they have to be a constant. Solving the second order ordinary differential equations (9.77) we obtain,

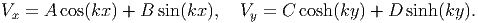V = A cos(kx) + B sin (kx ), V = C cosh(ky) + D sinh (ky). x y

(9.78)

Consistent with the boundary condition (9.75), we expect the functions V _x and V _y to be even functions, i.e., V _x(-x) = V _x(x) and V _y(-y) = V _y(y). Hence,

B = D = 0.

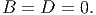(9.79)

Substituting (9.79) in the equation (9.78) and the result into equation (9.76) we obtain,

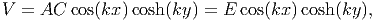V = AC cos(kx )cosh(ky) = E cos(kx )cosh(ky ),

(9.80)

where E is some arbitrary constant. The equation (9.80) on applying the boundary condition (9.75a), i.e., V (±b,y) = 0 yields that

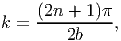(2n + 1)π k = ----------, 2b

(9.81)

where n is any integer. Since, the governing equation for V , (9.74) is a Laplace equation, it is a linear equation. Consequently, if two functions satisfy the given equation (9.74) then a linear combination of these functions also satisfies the Laplace equation. So the solution (9.80) can be written as,

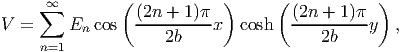∑∞ ( ) ( ) V = E cos (2n-+-1)πx cosh (2n-+-1)π-y , n 2b 2b n=1

(9.82)

where E_n’s are constant. Substituting (9.82) in the boundary condition (9.75b), i.e., V (x,±h) = x² - h² we obtain,

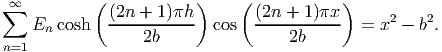∑∞ ( ) ( ) En cosh (2n-+-1)πh- cos (2n-+-1)πx- = x2 - b2. n=1 2b 2b

(9.83)

Now, we have to find the constants E_n such that for -b ≤ x ≤ b, a linear combination of cosines approximates the quadratic function on the right hand side of equation (9.83). Using the standard techniques in fourier analysis we obtain,

( ) ∫ b ( ) En cosh (2n-+-1)πh- = [x2 - b2]cos (2n-+-1-)πx- . 2b -b 2b

(9.84)

Integrating the above equation and rearranging, we obtain

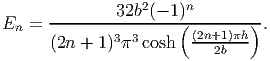2 n E = ---------32b-(- 1-)(------)-. n (2n + 1 )3π3 cosh (2n+1)πh- 2b

(9.85)

Substituting equation (9.85) in (9.82) and the resulting equation in (9.73), we obtain

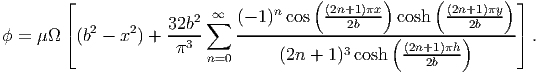⌊ ∞ n ( (2n+1)πx) ((2n+1)πy) ⌋ 2 2 32b2-∑ (--1)-cos-----2b----cosh-----2b---- ϕ = μΩ ⌈ (b - x ) + π3 3 ( (2n+1)πh) ⌉ . n=0 (2n + 1) cosh 2b

(9.86)

Having found the stress function, we are now in a position to find the shear stresses and the warping function. First, we compute the stresses as,

∂ϕ
σxz = ---
∂y ( ( ) [ ] ( ) )
2 { ∞ (- 1)ncos (2n+1)πx- (2n+1)π- sinh (2n+1-)πy }
= μΩ 32b-- ∑ --------------2b--------2b(--------)----2b---- ,
π3 ( 3 (2n+1)πh- )
n=0 (2n + 1) cosh 2b

(9.87)

σyz = - ∂ϕ-
(∂x ( ) [ ] ( ))
{ 2∑∞ (- 1)nsin (2n+1)πx- (2n+1)π cosh (2n+1)πy }
= μΩ 2x + 32b-- --------------2b--------2(b-------)----2b---- .
( π3 (2n + 1)3cosh (2n+1)πh- )
n=0 2b

(9.88)

Figure 9.16: Schematic of shear stress distribution in a bar with solid rectangular cross section subjected to end torsion

Figure 9.16 schematically shows the shear stress distribution in a rectangular cross section along the sides of the rectangle and along the lines x = 0 and y = 0. The shear stress is maximum on the boundary and zero at the center of the rectangle. The maximum shear stress occurs at the middle of the long side and is given by,

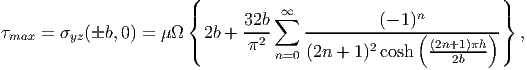({ ∞ )} 32b-∑ ---------(--1)n---------- τmax = σyz(±b, 0) = μΩ (2b + π2 2 ( (2n+1)πh) ) , n=0 (2n + 1) cosh 2b

(9.89)

where we have assumed that h ≥ b.

Substituting (9.87) in equation (9.45) and solving the first order ordinary differential equation for ψ we obtain

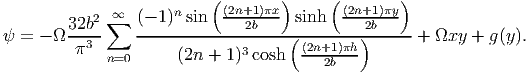( ) ( ) 2∑∞ (- 1)nsin (2n+1)πx- sinh (2n+1)πy ψ = - Ω32b-- --------------2b----(--------)2b-----+ Ωxy + g(y). π3 (2n + 1)3cosh (2n+1)πh- n=0 2b

(9.90)

Substituting equations (9.88) and (9.90) in equation (9.46) and solving the first order ordinary differential equation for g we obtain g(y) = D₀, a constant. Since, the bar does not move as a rigid body, we want ψ(0, 0) = 0. Hence, D₀ = 0. Consequently, the warping function is,

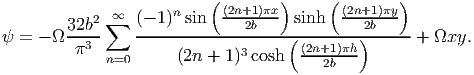( ) ( ) 2 ∞ (- 1)n sin (2n+1)πx- sinh (2n+1)πy ψ = - Ω 32b-∑ --------------2b---(--------)2b----+ Ωxy. π3 3 (2n+1-)πh- n=0 (2n + 1) cosh 2b

(9.91)

Figure 9.17: Warping deformation of a rectangular section due to end torsion

Figure 9.17 plots the warped rectangular section.

Substituting (9.87) and (9.88) in equation (9.57) we can relate the applied torque, T to the realized angle of twist per unit length, Ω as

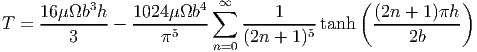3 4 ∞∑ ( ) T = 16μ-Ωb-h-- 1024μΩb--- ----1---- tanh (2n-+-1)πh- 3 π5 n=0(2n + 1)5 2b

(9.92)

This relation is expressed as

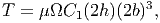3 T = μΩC1 (2h)(2b) ,

(9.93)

where C₁ is a non-dimensional parameter which depends on h∕b. In the same spirit, the maximum shear stress, (9.89) could be related to the applied torque as,

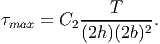T τmax = C2 --------2. (2h)(2b)

(9.94)

where C₂ is another non-dimensional parameter which depends on h∕b. Values of C₁ and C₂ for various h∕b ratios are tabulated in table 9.1.

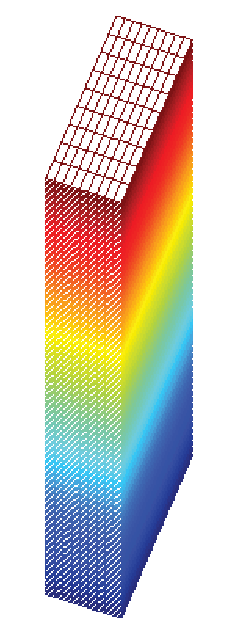(a) Undeformed rectangular bar 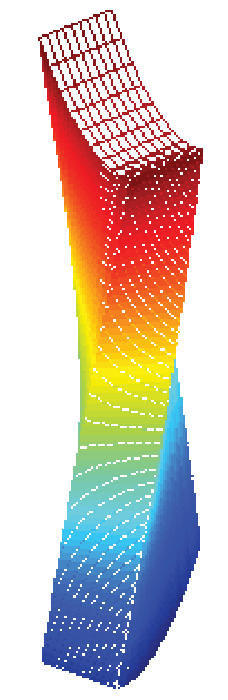(b) Deformed rectangular bar

Figure 9.18: A bar with rectangular cross section subjected to end twisting moment

Table 9.1: Tabulation of parameters C₁ and C₂ as a function of h∕b


h∕b	C₁	C₂

1	0.141	4.80

1.5	0.196	4.33

2.0	0.229	4.06

3.0	0.263	3.74

4.0	0.281	3.54

6	0.299	3.34

10	0.312	3.21

∞	0.333	3.00

Thus, for thin rectangular sections, when h∕b tends to ∞,

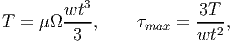3 T = μΩ wt--, τmax = 3T--, 3 wt2

(9.95)

where we have assumed the section to have a thickness, t = 2b and depth, w = 2h. It should be mentioned that irrespective of the orientation of the thin rectangular section, the equation (9.95) remains the same. This is because of the requirement that h ≥ b. Physically, irrespective of the orientation of the section, the torsion is resisted by the spatial variation of the shear stresses through the thickness of the section as shown in figure 9.19.

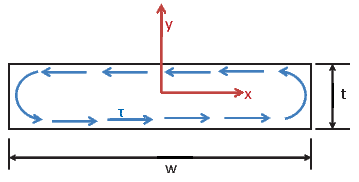(a) Thin rectangular section oriented along x direction 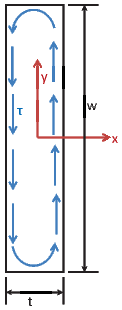(b) Thin rectangular section oriented along y direction

Figure 9.19: Schematic of shear stress distribution in a thin rectangular section in different orientations

9.3.3 Thin rolled section

Here we study the problem of end torsion of a bar whose cross section is narrow and has small curvature such as those shown in figure 9.20. Such sections are usually made of hot rolled steel and hence are called rolled sections. Since, the shape across the thickness is similar to that of the thin rectangular section, excepting at the bent corners, it is assumed that the solution obtained for thin rectangular section holds. In order for the corners not to change the displacement and stress field from that obtained for a thin rectangular section, it is required that the radius of curvature of these corners be large.

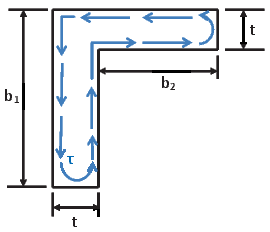(a) Angle section 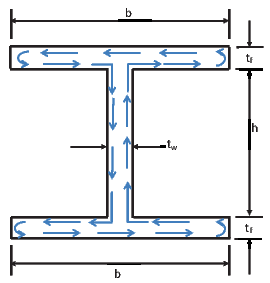(b) I section

Figure 9.20: Schematic of shear stress distribution in a thin rolled sections

Thus, for the angle section shown in figure 9.20a, the torque required to realize a given angle of twist per unit length and the maximum shear stress in the section is obtained from (9.95) as

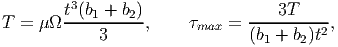t3(b1-+-b2) ----3T---- T = μΩ 3 , τmax = (b1 + b2)t2,

(9.96)

where we have treated the entire angle without the 90 degree bend, as a thin walled rectangular section. In order for the above equations to be reasonable, (b₁ + b₂)∕t > 10 and the radius of the curvature of the bent corner large.

Similarly, for the I section shown in figure 9.20b, the torque required to realize a given angle of twist per unit length and the maximum shear stress in the section is obtained from (9.95) as

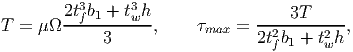2t3fb1 + t3wh 3T T = μΩ -----------, τmax = --2-----2--, 3 2tfb1 + twh

(9.97)

where the torsional stiffness due to each of the three plates - the two flange plates and the web plate - is summed algebraically. This is justified because the shear stress distribution in each of these plates is similar to that of a thin rectangular section subjected to torsion, as indicated in the figure 9.20b. However, comparison of this solution with the exact result for this geometry is beyond the scope of this notes.

9.3.4 Triangular cross section

Figure 9.21: Orientation of an equilateral triangle with respect to a given coordinate basis

Finally, we consider the torsion of a cylinder with equilateral triangle cross section, as shown in figure 9.21. The boundary of this section is defined by,

√ -- √ -- f(x,y ) = [x - 3y + 2a][x + 3y + 2a][x - a] = 0,

(9.98)

where a is a constant and we have simply used the product form of each boundary line equation. Assuming that the Prandtl stress function to be of the form,

√ -- √ -- ϕ = K [x - 3y + 2a][x + 3y + 2a][x - a],

(9.99)

so that the boundary condition (9.50) is satisfied. It can then be verified that the potential given in equation (9.99) satisfies (9.47) if

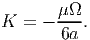μΩ- K = - 6a .

(9.100)

Substituting equation (9.99) in (9.57) and using (9.100) we obtain the torque to be

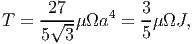27 3 T = -√--μ Ωa4 = -μΩJ, 5 3 5

(9.101)

where the polar moment of inertia for the equilateral triangle section, J = 3 √ --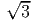
3 a⁴.

The shear stresses given in equation (9.44) evaluates to

μΩ- σxz = a [x - a]y, (9.102) μΩ σyz = ---[x2 + 2ax - y2], (9.103) 2a

on using equations (9.99) and (9.100). The magnitude of the shear stress at any point is given by,

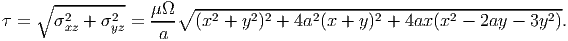∘ --------- μ Ω ∘ ---------------------------------------------- τ = σ2xz + σ2yz = -a- (x2 + y2)2 + 4a2(x + y)2 + 4ax (x2 - 2ay - 3y2).

(9.104)

Since, for torsion the maximum shear stress occurs at the boundary of the cross section, we investigate the same at the three boundary lines. We begin with the boundary x = a. It is evident from (9.102) that on this boundary σ_xz = 0. Then, it follows from (9.103) that σ_yz is maximum at y = 0 and this maximum value is 3aμΩ∕2. It can be seen that on the other two boundaries too the maximum shear stress, τ_max = 3aμΩ∕2.

Substituting (9.99) in equations (9.45) and (9.46) and solving the first order differential equations, we obtain the warping displacement as,

ψ = -Ω-y[3x2 - y2], 6a

(9.105)

on using the condition that the origin of the coordinate system does not get displaced; a requirement to prevent the body from displacing as a rigid body.

9.4 Twisting of hollow section

In this section, as in the previous, we consider a bar subjected to twisting moments at its ends. The bar axis is straight and the shape of the cross section is constant along the axis. The bar is assumed to have a hollow cross section, i.e, a multiply connected domain. That is any closed curves in the cross section cannot be shrunk to a point in the domain itself without leaving the domain.

Figure 9.22: Example of a multiply connected cross section. Elliptical cross section with two circular holes.

Let us assume that the boundary of the cross section is defined by a function, f(x,y) = 0, the set of points constituting this boundary by ∂_o, and the enclosed area by A_o. The boundary of the i^th void in the interior of the cross section is defined by the function g_i(x,y) = 0, the set of points constituting this boundary of the i^th void by ∂_i and the area enclosed by the void, A_i. Thus, the area of the cross section, A_cs is given by

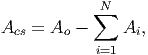∑N A = A - A , cs o i i=1

(9.106)

where we have assumed that there are N voids.

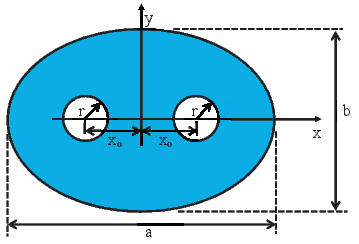
Thus, for the cross section shown in figure 9.22, with the boundary of the cross section in the form of an ellipse centered about origin and oriented such that the major and minor axis coincides with the e_x and e_y directions respectively and having two circular shaped voids of radius, r centered at (±x_o, 0), the functions f(x,y) and g_i(x,y) would be: f(x,y) = x²∕a² + y²∕b² - 1, g₁(x,y) = (x - x_o)² + y² - r² and g₂(x,y) = (x + x_o)² + y² - r². Consequently, this body is assumed to occupy a region in Euclidean point space, defined by = {(x,y,z)|f(x,y) ≤ 0, 0 ≤ Z ≤ L}-{(x,y,z)|f(x,y) ≤ 0, 0 ≤ Z ≤ L}- {(x,y,z)|f(x,y) ≤ 0, 0 ≤ Z ≤ L}-{(x,y,z)|g₁(x,y) ≤ 0, 0 ≤ Z ≤ L}- {(x,y,z)|f(x,y) ≤ 0, 0 ≤ Z ≤ L}-{(x,y,z)|g₂(x,y) ≤ 0, 0 ≤ Z ≤ L}, where L is a constant.

Since, we are interested in the case where in the bar is subjected to pure end torsional moment, the traction boundary conditions are:

t(en)(∂Ao, z ) = o, (9.107) t (∂A ,z ) = o, (9.108) ∫ (emi) i t (x,y,0)da = o, (9.109) a (-ez) ∫ t(ez)(x,y, L)da = o, (9.110) ∫ a (xex + yey) ∧ t(-ez)(z,y,0)da = - Tez, (9.111) ∫a (xex + yey) ∧ t(e )(x,y, L)da = T ez, (9.112) a z

where

[∂∂fyex - ∂∂fxey] [∂g∂iy ex - ∂∂gxiey] en = ∘----------(--)--, emi = ∘----------(---)-, (∂f)2 + ∂f 2 (∂gi)2 + ∂gi 2 ∂x ∂y ∂x ∂y

(9.113)

and T is a constant. Here e_n is obtained such that it is in the xy plane, perpendicular to grad(f) and is a unit vector. Similarly, e_{m_i} is obtained such that it is in the xy plane, perpendicular to grad(g_i) and is a unit vector. Recognize that grad(f) gives the tangent vector to the cross section for any point in ∂_o and grad(g_i) is the tangent vector to the cross section at any point in ∂_i. Further, since one end of this body is assumed to be fixed against twisting but free to displace axially and the other end free to displace in all directions, the displacement boundary conditions for this problem is,

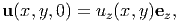u(x,y, 0) = uz(x,y)ez,

(9.114)

Thus, we find that the boundary value problem is similar to that in the previous section (section 9.3) except for the additional boundary condition (9.108). Hence, we assume that the stress is as given in equation (9.44), in terms of the Prandtl stress function, ϕ = 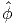ˆ
ϕ (x,y). Following the standard procedure, as outlined in section 9.3, it can be shown that the stress function should satisfy,

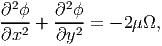∂2ϕ ∂2ϕ ---2 + --2-= - 2μ Ω, ∂x ∂y

(9.115)

where μ is the shear modulus, and Ω is the angle of twist per unit length.

For the assumed stress state, (9.44), the boundary condition (9.107) requires that grad(ϕ) ⋅ grad(f) = 0 on ∂_o. Thus,

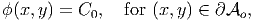ϕ(x, y) = C0, for (x,y) ∈ ∂Ao,

(9.116)

where C₀ is a constant. As before, since, the stress and displacement fields depend only in the derivative of the stress potential and not its value at a location, it suffices to find this stress function up to a constant. Therefore, we arbitrarily set C₀ = 0, understanding that it can take any value and that the stress and displacement field would not change because of this. Hence, equation (9.116) reduces to requiring,

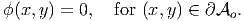ϕ(x, y) = 0, for (x, y) ∈ ∂Ao.

(9.117)

The boundary condition (9.108) requires that grad(ϕ) ⋅ grad(g_i) = 0 on ∂_i. Hence,

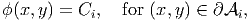ϕ (x,y) = C , for (x,y) ∈ ∂A , i i

(9.118)

where C_i’s are constants. Though ϕ is a constant over all free surfaces, the value of this constant can differ between distinct free surfaces, i.e., ∂_i. Also, recognize that these constants cannot be set to zero, as we have already set C₀ = 0.

The boundary condition (9.109) and (9.110) for the stress state (9.44) require

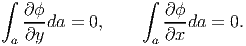∫ ∫ ∂ϕ- ∂-ϕ a ∂yda = 0, a∂x da = 0.

(9.119)

Appealing to Green’s theorem for multiply connected domains, (2.278) and (2.279), the above equation (9.119) reduces to

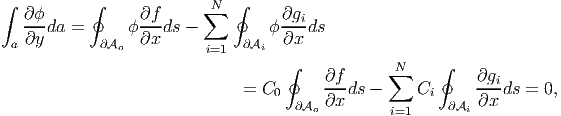∫ ∂ϕ ∮ ∂f ∑N ∮ ∂gi
---da = ϕ --ds - ϕ---ds
a ∂y ∂Ao ∂x i=1 ∂Ai ∂x
∮ N∑ ∮
= C0 ∂f-ds - Ci ∂gids = 0,
∂Ao ∂x i=1 ∂Ai ∂x

(9.120)

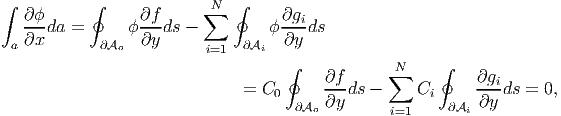∫ ∂ϕ ∮ ∂f ∑N ∮ ∂gi
---da = ϕ --ds - ϕ---ds
a ∂x ∂Ao ∂y i=1 ∂Ai ∂y
∮ N∑ ∮
= C ∂f-ds - C ∂gids = 0,
0 ∂Ao ∂y i ∂Ai ∂y
i=1

(9.121)

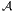where we have used (9.116) and (9.118) and the fact that ∮ _{∂_o}grad(f)ds = 0, ∮ 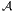_{∂_i}grad(g_i)ds = 0.

Finally, the boundary conditions (9.111) and (9.112) for the stress state (9.44) yields,

∫ [ ∂ ϕ ∂ ϕ] - x--- + y--- da = T. a ∂x ∂y

(9.122)

Noting that

[ ]
∫ ∂ ϕ ∫ ∂(x ϕ) ∮ ∂f N∑ ∮ ∂gi ∫
x--- = ------ - ϕ da = xϕ ---ds- xϕ ---ds - ϕda
a ∂x a ∂x ∂Ao ∂y i=1 ∂Ai ∂y a
∑N ∮ ∫ ∑N ∫
= - Ci x∂gids - ϕda = - CiAi - ϕda,
i=1 ∂Ai ∂y a i=1 a

(9.123)

∫ ∂ϕ ∫ [∂(yϕ ) ] ∮ ∂f ∑N ∮ ∂gi ∫
y--- = ------- ϕ da = yϕ---ds - yϕ----ds - ϕda
a ∂y a ∂y ∂Ao ∂x i=1 ∂Ai ∂x a
∑N ∮ ∫ ∑N ∫
= - C y∂gids - ϕda = - C A - ϕda,
i ∂Ai ∂x a i i a
i=1 i=1

(9.124)

where we have used the Green’s theorem for multiply connected domain (2.278) and (2.279) and equations (9.117) and (9.118). Recognize that from Green’s theorem,

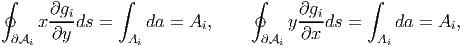∮ ∫ ∮ ∫ ∂gi- ∂gi- x∂y ds = da = Ai, y ∂x ds = da = Ai, ∂Ai Ai ∂Ai Ai

(9.125)

where A_i is the area enclosed by the void.

Substituting the above equations (9.123) and (9.124) in (9.122) we obtain,

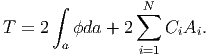∫ N ∑ T = 2 ϕda + 2 CiAi. a i=1

(9.126)

Thus, we have to find ϕ such that the governing equation (9.115) has to hold along with the boundary conditions (9.117) and (9.118). Then, we use equation (9.126) to find the torsional moment required to realize a given angle of twist per unit length, Ω.

9.4.1 Hollow elliptical section

Figure 9.23: Hollow elliptical section

Here we study the torsion of a bar with a hollow elliptical section as shown in figure 9.23 where the inner boundary is a scaled ellipse similar to that of the outer boundary. Since, the inner ellipse is a scaled outer ellipse, the Prandtl stress function obtained for solid ellipse cross section in section 9.3.1 is a constant at the inner surface. Hence, the boundary condition (9.118) holds and therefore this stress function

[ ] --a2b2- x2- y2- ϕ = μΩ a2 + b2 a2 + b2 - 1 ,

(9.127)

is a solution to this boundary value problem of twisting of a hollow elliptical bar. Now, the constant C₁, the value of ϕ at the inner boundary is obtained as,

--a2b2- [ 2 ] C1 = μΩ a2 + b2 k - 1 .

(9.128)

Using (9.127) and (9.128) in (9.126), twisting moment is obtained as

3 3 T = μΩ π--a-b--[1 - k4]. a2 + b2

(9.129)

Since, the stress function is same as in the case of a solid elliptical section, the stress distribution is also identical as before. Therefore, the maximum shear stress occurs at x = 0, y = ±b and is given by,

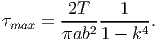-2T----1---- τmax = πab2 1 - k4.

(9.130)

Before concluding this section we observe that this solution scheme can be applied to other cross sections whose inner boundary coincides with a contour line of the stress function in the corresponding solid section problem.

9.4.2 Thin walled tubes

In this section, we seek approximate solution to the case when, the bar, in the form of a thin walled tube is subjected to end torsion. We do not assume that the cross section of the tube to be of any particular shape. While we assume that the thickness of the cross section, t is small, we do not assume that it is uniform. Let the boundary of the cross section be defined by a function, f(x,y) = 0, the set of points constituting this boundary be denoted by ∂_o, and the enclosed area by A_o. The boundary in the interior of the cross section is defined by the function g₁(x,y) = 0, the set of points constituting this boundary is denoted by ∂_i and the area enclosed by, A_i. Then the area of the cross section is A_cs = A_o - A_i.

Figure 9.24: Thin walled section

In order to satisfy the requirement that the lateral surface is taction free, boundary conditions (9.107) and (9.108), the stress function has to be zero at the outer surface (9.117) and should be a constant at the inner surface (9.118). Hence, we assume that the stress function varies linearly through the thickness of the section. This assumption would be reasonable given that the thickness of the section is small. Thus,

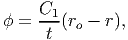C ϕ = -1(ro - r), t

(9.131)

where C₁ is a constant, the value of ϕ at the inner surface, r_o is the radial distance of the outer boundary from the centroid of the cross section, r is the radial distance of any point in the cross section. If r_i is the radial distance of the inner boundary from the centroid of the cross section, then t = r_o - r_i.

For the warping displacement, ψ to be single valued in the thin walled tube shown in figure 9.24,

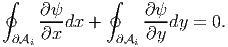∮ ∮ ∂ψ-dx + ∂ψ-dy = 0. ∂Ai ∂x ∂Ai ∂y

(9.132)

Substituting equations (9.45) and (9.46) in the above equation,

∮ [ ] ∮ [ ] 1-∂ϕ- 1-∂ϕ- ∂A μ ∂y + Ωy dx - ∂A μ ∂x + Ωx dy = 0. i i

(9.133)

Using Green’s theorem (2.271) we find that

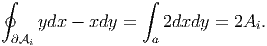∮ ∫ ydx - xdy = 2dxdy = 2Ai. ∂Ai a

(9.134)

Since, the stress function is assumed to vary linearly over the domain, grad(ϕ) = -C₁∕te_r, where e_r is a unit vector normal to the boundary of the cross section. Consequently,

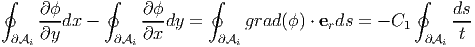∮ ∮ ∮ ∮ ∂-ϕdx - ∂-ϕdy = grad (ϕ ) ⋅ e ds = - C ds- ∂Ai∂y ∂Ai∂x ∂Ai r 1 ∂Ai t

(9.135)

Substituting equations (9.134) and (9.135) in (9.133) we obtain

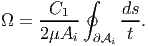∮ Ω = -C1-- ds. 2μAi ∂Ai t

(9.136)

Since, ϕ varies linearly through the thickness of the cross section, using trapezoidal rule for integration we find that

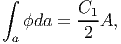∫ C1- ϕda = 2 A, a

(9.137)

where A is the area of the cross section. Here it should be pointed out that trapezoidal rule for integration gives the exact value when the variation is linear. Now, substituting equation (9.137) in (9.126) we obtain the torque to be,

[ A ] T = C1A + 2C1Ai = 2C1 Ai + -- = 2C1Ac, 2

(9.138)

where A_c is the area enclosed by the centerline of the cross section. As a consequence of the section being thin walled, A_i ≈ A_c. Therefore, from equations (9.136) and (9.138) we obtain,

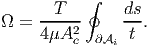T ∮ ds Ω = ----2- ---. 4μA c ∂Ai t

(9.139)

Here it should be recollected that the thickness of the section can vary along the circumference of the cross section.

Finally, we estimate the shear stress in the cross section. Since, the shear stress in the cross section is equal to the magnitude of grad(ϕ), we obtain

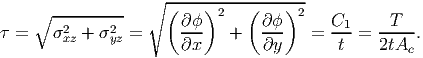∘ (----)-----(---)-- ∘ --------- ∂ ϕ 2 ∂ ϕ 2 C1 T τ = σ2xz + σ2yz = --- + --- = ---= -----. ∂x ∂y t 2tAc

(9.140)

Thus, τt is constant throughout the section.

9.5 Summary

In this chapter, we obtained the stress and displacement fields in a straight, prismatic bar subjected to end twisting moments. For the case of thick walled closed sections and thin walled sections of any shape, we obtained an expression relating the torque with the angle of twist per unit length and the shear stress with the torque. However, for thick walled open sections, a procedure was outlined to obtain the stress and displacement fields and the same illustrated for elliptical, rectangular and triangular shaped cross sections. In all these cases, the section was assumed to be free to warp, so that no warping stresses develop. Generalization for the case when warping is restrained, is not straight forward and is beyond the scope of this lecture notes.

9.6 Self-Evaluation

Knowing that the inner diameter of an annular cylinder is d_i = 30 mm and that its outer diameter is d_o = 40 mm, determine the torque that causes a maximum shearing stress of 52 MPa in the annular cylinder.
Neglecting the effect of stress concentration, determine the largest torque that may be applied at A in the composite bar shown in figure 9.25. Assume that the end C is fixed against twisting and the allowable shear stress is 104 MPa in the 55 mm diameter solid steel rod BC and 55 MPa in the 40 mm diameter solid brass rod AB.

Figure 9.25: Figure for problem 2

Figure 9.26: Figure for problem 3
The composite shaft shown in figure 9.26 is to be twisted by applying a torque T at end A. Knowing that the shear modulus for steel is 77 GPa and for brass is 37 GPa, determine the largest angle through which end A may be rotated if the following allowable stresses are not to be exceeded 100 MPa in steel and 50 MPa in brass.
The stepped circular shaft, shown in figure 9.27, is rigidly attached to a wall at E. The diameter of the shaft AC, d_AC is 25mm and that of the shaft CE, d_CE is 50mm. Determine the angle of twist of the end A when the torques at B and D are applied as shown in the figure 9.27. Let T_b = 150 Nm and T_d = 1000 Nm. Assume the shear modulus G = 80 GPa. Neglect stress concentration effects.

Figure 9.27: Figure for problem 4

Figure 9.28: Figure for problem 5
(a) Determine the reactions for the circular stepped shafts shown in figure 9.28. Let the diameter of the shaft AC, d_AC be 25mm and that of the shaft CE, d_CE be 50mm and the torques, T_b = 400 Nm and T_d = 2000 Nm. (b) Plot the angle of twist diagram for the shaft along its length. The material parameters for the material with which this shaft is made are: Young’s modulus, E = 120 GPa and Poisson’s ratio, ν = 1/3. Neglect stress concentration effects.
Same torque is applied to two hollow shafts of same length 0.5 m and made of same material but having cross sections in the shape of T and C, as shown in figure 9.29. Neglecting the effect of stress concentration, compare the maximum shearing stress that occurs in these sections and the angle of twist of the shaft. Assume, t_w = t = 6 mm, t_f = 12 mm, b = 25 mm, h = 30 mm. Recognizing that the area of both the cross sections is same, comment on which section is economical.

(a) T section (b) C section

Figure 9.29: Figure for problem 6

Figure 9.30: Circular shaft centered about (a, 0) with a keyway. Figure for problem 7
Verify that the admissibility of the following Prandtl stress function for a circular shaft with a keyway, as shown in figure 9.30,
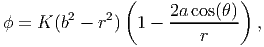$( ) 2 2 2a-cos(θ) ϕ = K (b - r ) 1 - r ,$

where K is a constant. If the stress function is admissible,
- Find the constant K
- Compute the Cartesian components of the shear stress, σ_xz and σ_yz
- Show that the resultant shear stresses on the shaft and the keyway boundaries are given by
  $[ ] -----b2---- τshaft = μΩa 4a2 cos2(θ) - 1 , τkeyway = μ Ω[2a cos(θ) - b],$
  
  where Ω is the angle of twist per unit length and μ is the shear modulus.
- Determine the maximum value of the shear stress in the shaft and keyway
- Show that the maximum value of the keyway shear stress is approximately twice that of the shear stress in the shaft
- Determine the maximum shear stress for a solid shaft of circular section of radius a, τ_max^solidshaft.
- Plot the τ_max^keyway∕τ_max^solidshaft, where τ_max^keyway is the maximum shear stress in the keyway, for 0 ≤ b∕a ≤ 1 assuming same torque is applied on both the sections. Show that τ_max^keyway∕τ_max^solidshaft → 2 as b∕a → 0. This shows that a small notch would result in the doubling of the shear stresses in the circular section under same torsion.
- Show that the warping function for the shaft with a keyway is
  $[ 2 ] ψ = - μΩ- x2 + y2 - 2ax + -2b-ax--- b2 , 2 x2 + y2$
  
  where x and y are the coordinates of a typical point in the cross section.
Two tubular thin walled sections shown in figure 9.31 have the same wall thickness t and the same perimeter and hence b = aπ∕2. Neglecting the stress concentration, find the ratio of the shear stresses if,
- Both the sections are subjected to equal twisting moment
- Both the sections have the same angle of twist per unit length
Comment on which of these sections is economical.
Two sections, a solid circular section and a hollow rectangular tube as shown in figure 9.32 are subjected to the same torque, T. If the two tubes are of same length, L and made of the same material and differ only in the shape of the cross section, find the thickness of the rectangular section so that (a) the maximum shear stresses is same in both the sections (b) angle of twist per unit length is same for these two sections. Comment on which of these sections is economical.
During the early development of the torsion formulation, Navier assumed that there is no warping displacement for any cross section. Show that although such an assumed displacement field will satisfy all the required governing differential equations, it would not satisfy the boundary conditions and hence this is not an acceptable solution.

(a) Annular cylinder (b) Annular square

Figure 9.31: Sections for problem 8

(a) Annular rectangular (b) Solid circular

Figure 9.32: Sections for problem 9