
In some applications such as rail tracks, the member subjected to loads is supported on continuous foundations. That is the reactions due to external loading is distributed along the length of the member. Here we study on how to get the stresses and displacements in these members resting on continuous foundations. If the dimensions of this member is such that, it is longer along one of the axis, called the longitudinal axis in comparison with the dimensions along the other directions, it is called as a beam. If we assume that the reaction force offered by the continuous support is a function of the displacement that of the member, the support is called as elastic. A beam resting on an elastic support is said to be beam on elastic foundation.
In this chapter, we first formulate this problem of beam on an elastic foundation for a general loading condition. Then, we study the problem of a concentrated load at the mid point of a beam that is infinitely long. Appealing to the principle of superposition we obtain the solution to the problem of a concentrated moment at mid span and uniformly distributed load of length L, centered about the midpoint of the beam.
In this section, we formulate the boundary value problem of beam on an elastic foundation. A beam having some cross section, resting on an elastic support is shown in figure 11.1. We assume that the reaction offered by the support at any point is directly proportional to the displacement of that point along the y direction and is in a direction opposite to the displacement. Thus, if Δ is the vertical displacement of a point in the beam, qy the support reaction per unit width of the beam, then the above assumption that the reaction force is proportional to the displacement mathematically translates into requiring
 | (11.1) |
Assuming the beam to be homogeneous, we obtained the equation (8.41) which we document here again:
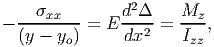 | (11.2) |
where yo is the y coordinate of the centroid of the cross section which can be taken as 0 without loss of generality provided the origin of the coordinate system used is located at the centroid of the cross section, E is the Young’s modulus, (x,y) is the coordinate of the point along the axis of the beam direction and the y direction, Mz is the z component of the bending moment, Izz is the moment of inertia of the section about the z axis.
In section 8.1, we integrated the equilibrium equations and obtained equations (8.18) and (8.25) which we record here:
where V y is the shear force along the y direction and qy is the transverse loading along the y direction. Combining the equations (11.3) and (11.4) we obtain,
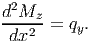 | (11.5) |
Substituting equation (11.2) in equation (11.5), we obtain
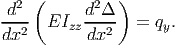 | (11.6) |
Assuming the beam to be homogeneous and prismatic, so that EIzz is constant through the length of the beam, and substituting equation (11.1) in equation (11.6), we obtain
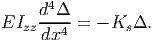 | (11.7) |
Defining,
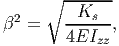 | (11.8) |
equation (11.7) can be written as
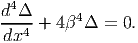 | (11.9) |
The differential equation (11.9) has a general solution:
![Δ = exp(- βx)[C1 sin (βx )+ C2 cos(βx )]+ exp (βx )[C3sin(βx )+ C4 cos(βx )],](main1417x.png) | (11.10) |
where Ci’s are constant to be determined from the boundary conditions.
Having found the deflection, the stress is estimated from (11.2) as
![d2 Δ
σxx = - E (y - yo)---2
dx 2
= - 2yE β {exp (- βx )[C2 sin(βx ) - C1 cos(βx )]
+ exp(βx)[C3 cos(βx) - C4 sin (βx)]},](main1418x.png) | (11.11) |
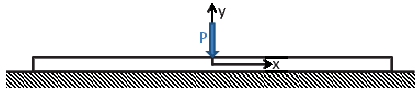
The first boundary value problem that we study for the beam on elastic foundation is when it is subjected to a point load at its mid span as shown in figure 11.2. The origin of the coordinate system is assumed to coincide with the point of application of the load. The beam length is assumed to be large enough compared to its lateral dimension that it can be considered to be infinitely long. (We shall quantify what length could be considered as infinitely long after we obtain the solution.)
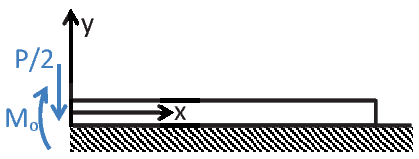
To obtain the solution we section the beam at x = 0, the point of application of the concentrated load, as shown in figure 11.3. Since, there is a concentrated force acting at x = 0, the shear force would be discontinuous at x = 0 and hence the fourth derivative of the deflection, Δ does not exist. Consequently, the governing equation (11.9) is valid only in the domain x > 0 and x < 0 and not at x = 0. Therefore, we segment the beam at x = 0 and solve (11.9) on each of the segments. Then, we ensure, the differentiability of the second order derivative of deflection so that the third order derivative exist. This is required to ensure the existence of shear force at x = 0.
We also expect the deflection to be symmetric about x = 0 that is, Δ(x) = Δ(-x) and therefore the slope of the deflection should be zero at x = 0, i.e.,
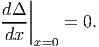 | (11.12) |
By sectioning the beam at x = 0 we find the bending moment and shear force at this location. Using equation (11.2) we find that
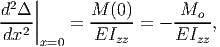 | (11.13) |
where Mo is the bending moment at x = 0, acting as shown in the figure 11.3 and
the negative sign is to account for the fact that it is hogging. Since, shear force
should exist, the continuity of the bending moment and 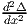 has to be ensured.
Therefore the value of bending moment at both the segments of the beam should
be the same.
has to be ensured.
Therefore the value of bending moment at both the segments of the beam should
be the same.
Substituting equation (11.2) in (11.3) and assuming the beam to be homogeneous and prismatic, we obtain
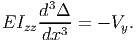 | (11.14) |
Since, there is a concentrated force at x = 0, V y(0+) = -V y(0-) and the equilibrium of an infinitesimal element centered about x = 0 requires that V y(0+) - V y(0-) = P. Hence, V y(0+) = P∕2 and V y(0-) = -P∕2. Thus, from equation (11.14) we obtain,
Further, we require that
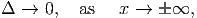 | (11.17) |
since, we expect the effect of the load would be felt only in its vicinity.
To obtain the solution, we first focus on the right half of the beam wherein x > 0. Then, the requirement (11.17) implies that the constants C3 and C4 in the general solution (11.10) has to be zero; otherwise Δ →∞ as x →∞. Next, the condition (11.12) requires that C1 = C2 = C0. Finally, the equation (11.15) tells us that
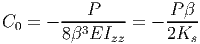 | (11.18) |
where to obtain the last equality we have made use of (11.8). Thus, in the domain, x > 0,
![P β
Δ = - ----exp (- βx )[cos(βx ) + sin(βx )].
2Ks](main1428x.png) | (11.19) |
Now, we consider the left half of the beam, i.e., x < 0. The requirement (11.17) implies that the constants C1 and C2 in the general solution (11.10) has to be zero. Next, the condition (11.12) requires that C3 = -C4 = C5. Finally, the equation (11.16) tells us that
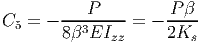 | (11.20) |
where to obtain the last equality we have made use of (11.8). Thus, in the domain, x < 0,
![P-β-
Δ = - 2K exp (βx )[cos(βx ) - sin (βx )].
s](main1430x.png) | (11.21) |
Thus, the distribution of the reaction from the foundation along the axis of the beam given in (11.1) evaluates to:
![{
P-βexp (- βx )[cos(βx ) + sin(βx )] x > 0
qy = - Ks Δ = P2β .
2 exp (βx)[cos(βx) - sin (βx )] x < 0](main1431x.png) | (11.22) |
The variation of the bending moment along the axis of the beam obtained from (11.2) is:
![d2 Δ { - -P exp(- βx)[cos(βx) - sin(βx)] x ≥ 0
Mz = EIzz---- = 4βP .
dx2 - 4β exp(βx )[cos(βx ) + sin(βx )] x ≤ 0](main1432x.png) | (11.23) |
The shear force variation along the axis of the beam computed using (11.14) is:
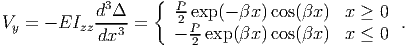 | (11.24) |
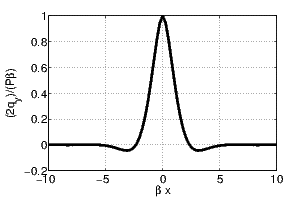 (a) Variation of support reaction
(a) Variation of support reaction
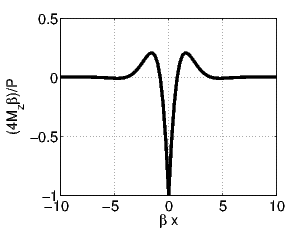 (b) Variation of bending moment
(b) Variation of bending moment
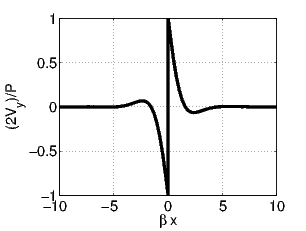 (c) Variation of shear force
(c) Variation of shear force
In figure 11.4 we plot the variation of the support reaction, bending moment and shear force along the axis of the beam. It can be seen from the figure that though the beam is assumed to be infinitely long the reaction force, bending moment and shear forces all tend to zero for βx > 5. Hence, a beam may be considered as long if its length is greater than, 5∕β. It can also be seen from the figure that the maximum deflection, support reaction, bending moment and shear occurs at z = 0 and these values are,
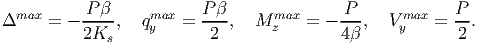 | (11.25) |
It can be seen from figure 11.4a that the support reaction changes sign. The support reaction changes sign at a point when qy = 0, i.e., sin(βx) = - cos(βx) or at x = 3π∕(4β). Since, the support reaction is proportional to the deflection, Δ, this change in sign of the support reaction also tells us that the beam will uplift at x = ±3π∕(4β). Hence, the beams have to be adequately clamped to the foundation to prevent it from uplifting.
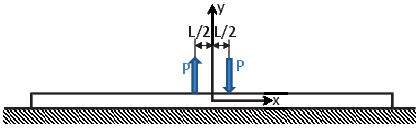
The concentrated moment, Mo, is considered to be equivalent to the action of two concentrated forces, P, equal in magnitude but opposite in direction and separated by a distance L as shown in the figure 11.5. Thus,
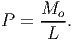 | (11.26) |
We obtain the solution to this loading case by superposing the displacement field obtained in the above example for a single point load. Thus, it follows from equations (11.17) and (11.19) that the displacement due to the downward acting force at a distance, L∕2 from the origin is,
![(| - -Pβ exp(- β(x - L ∕2))[cos(β (x - L∕2))
|{ 2Ks
ΔL ∕2 = Pβ + sin(β(x - L ∕2))] x ≥ L ∕2 .
||( - 2Ks exp(β(x - L ∕2))[cos(β (x - L∕2))
- sin(β(x - L ∕2))] x ≤ L ∕2](main1440x.png) | (11.27) |
Similarly, the displacement due to the upward acting force at a distance, -L∕2 from the origin is
![( -Pβ
||{ 2Ks exp(- β(x + L ∕2))[cos(β (x + L ∕2))
Δ = + sin (β(x + L∕2 ))] x ≥ - L∕2 .
-L∕2 || P2Kβs exp(β(x + L ∕2))[cos(β (x + L ∕2))
( - sin (β(x + L∕2 ))] x ≤ - L∕2](main1441x.png) | (11.28) |
Since, the displacement is small and the material obeys Hooke’s law, we can superpose the solutions as discussed in section 7.5.2. Hence, the displacement under the action of both the forces, Δ = ΔL∕2 + Δ-L∕2 evaluates to,
![(
| Mo-β-{exp(- β(x + L ∕2))[cos(β(x + L∕2 ))
||| 2KsL
|||| + sin(β(x + L ∕2))] - exp(- β(x - L ∕2))
||| [cos(β(x - L ∕2)) + sin(β (x - L∕2))]} x ≥ L ∕2
||{ M2oK-βL-{exp(- β(x + L ∕2))[cos(β(x + L∕2 ))
Δ = s + sin (β(x + L∕2 ))] - exp(β(x - L ∕2)) ,
||
||| M [cβos(β(x - L ∕2)) - sin(β (x - L∕2))]} - L∕2 ≤ x ≤ L∕2
|||| 2KosL-{exp(β(x + L ∕2))[cos(β(x + L∕2 ))
||| - sin (β(x + L∕2 ))] - exp(β(x - L ∕2))
|( [cos(β(x - L ∕2)) - sin(β (x - L∕2))]} x ≤ - L∕2](main1442x.png) | (11.29) |
where we have used equation (11.26). When L → 0 and PL → Mo the above equation (11.29) evaluates to,
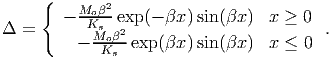 | (11.30) |
Having found the displacement, (11.30), the variation of the bending moment along the axis of the beam obtained from (11.2) is:
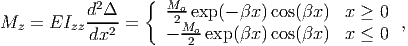 | (11.31) |
and the shear force variation computed using (11.14) is:
![3 { Moβ
V = - EI d-Δ- = -2--exp(- βx )[cos(βx ) + sin(βx )] x ≥ 0 .
y zzdx3 Mo2β-exp(βx )[cos(βx ) - sin(βx )] x ≤ 0](main1445x.png) | (11.32) |
Next, we study the problem of an infinite beam on an elastic foundation subjected to a uniformly distributed load of length L symmetrically on either side of the origin, as shown in figure 11.6. As before, we apply the principle of superposition to find the deflection at a point to be
![( ∫L∕2
|| - 2βKs -L∕2exp (- β (x - a))[cos(β (x - a))
||| + sin(β (x - a ))]qda x ≥ L ∕2
|||| β {∫ x
||| - 2Ks - L∕2exp(- β(x - a )) [cos(β (x - a ))
{ + sin(β(x - a))]qda
Δ = | ∫ L∕2 .
||| + x exp(β(x - a )) [cos(β (x - a ))
||| - sin(β(x - a ))]qda } - L ∕2 ≤ x ≤ L∕2
|||| - -β-∫ L∕2 exp(β (x - a))[cos(β (x - a))
|( 2Ks - L∕2
- sin(β (x - a ))]qda x ≤ - L∕2](main1447x.png) | (11.33) |
Evaluating the integrals in equation (11.33) we obtain
![(| - -q-exp(- β (x + L ∕2))[- cos(β(L ∕2 + x))
||| 2Ks
||{ -q- + cos(β(L ∕2 - x))exp (βL)] x ≥ L ∕2
Δ = - 2Ks {2 - cos(β(L∕2 - x ))exp(- β(L ∕2 - x)) .
|| - cos(β(L ∕2 + x))exp(- β(L ∕2 + x))} - L∕2 ≤ x ≤ L∕2
||| - -q- exp(β(x - L ∕2))[- cos(β(L ∕2 - x))
|( 2Ks + cos(β(L ∕2 + x))exp (βL)] x ≤ - L∕2](main1448x.png) | (11.34) |
Having found the displacement, (11.34), the variation of the bending moment along the axis of the beam obtained from (11.2) is:
![( q
|| - 4β2 exp (- β (x + L∕2))[- sin(β(x + L ∕2))
||| sin(β(L ∕2 - x))exp (βL)] x ≥ L ∕2
|{ - -q2 [sin (β(L∕2 - x ))exp(- β(L ∕2 - x))
Mz = 4β ,
||| +qsin(β (L∕2 + x))exp (- β(L∕2 + x ))] - L∕2 ≤ x ≤ L∕2
|||( - 4β2 exp (β (x - L∕2)) [sin(β(L ∕2 - x))
+ sin(β(L ∕2 + x))exp (βL)] x ≤ - L∕2](main1449x.png) | (11.35) |
and the shear force variation computed using (11.14) is:
![( -q
||| 4β exp(- β(x + L ∕2)){sin(β(L ∕2 + x))
||| - cos(β(L ∕2 + x))
|||| + [sin(β (L∕2 - x)) + cos(β(L ∕2 - x))]exp(βL )} x ≥ L ∕2
||| -q {exp(- β(L ∕2 - x))[cos(β(L∕2 - x ))
||{ 4β
V = - sin (β (L∕2 - x))] .
y || - exp (- β (L ∕2 + x))[cos(β(L ∕2 + x))
||| - sin(β (L∕2 + x))]} - L∕2 ≤ x ≤ L∕2
|||| -q exp(β(x - L ∕2)){sin(β(L ∕2 - x))
||| 4β
||| - cos(β(L ∕2 - x))
( + [sin (β (L∕2 + x)) + cos(β(L ∕2 + x))]exp(βL )} x ≤ - L∕2](main1450x.png) | (11.36) |
In this chapter, we formulated and solved the problem of a concentrated load acting on a long beam on elastic foundation. Using this solution and appealing to the principal of superposition, we solved two problems. One of the problems is that of a concentrated moment on a long beam on elastic support. The other problem is that of uniformly distributed load of length L, on a long beam continuously supported at the bottom. These problem serve as an illustration of the use of principle of superposition.
Find the length of the beam beyond which it would require clamping to prevent uplift.