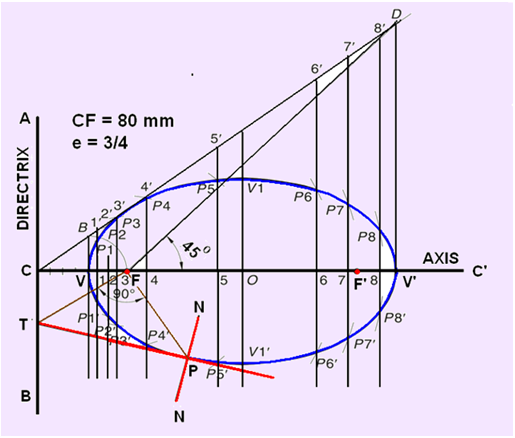
Figure 5. drawing an ellipse if the distance of focus from the directrix and the eccentricity is given                 

5.With F as a centre and radius = 1–1’, cut two arcs on the perpendicular through 1 to locate P1 and P1’. Similarly, with F as centre and radii = 2–2’, 3–3’, etc., cut arcs on the corresponding perpendiculars to locate P2 and P2’, P3 and P3’, etc. Also, cut similar arcs on the perpendicular through O to locate V1 and V1’.

6.Draw a smooth closed curve passing through V, P1, P/2, P/3, …, V1, …, V’, …, V1’, … P/3’, P/2’, P1’.

7.Mark F’ on CC’ such that V’ F’ = VF.

An ellipse is also the set of all points in a plane for which the sum of the distances from the two fixed points (the foci) in the plane is constant.This is clear from figure 6.

 

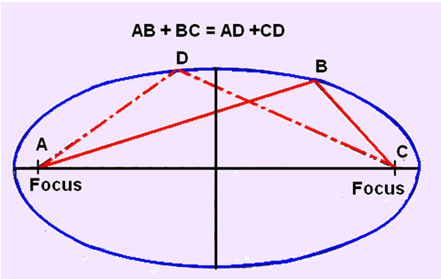
Figure 6.Another definition of ellipse