Ellipse

Referring to figure 4, an ellipse can be defined in the following ways.

• An ellipse is obtained when a sectio plane, inclined to the axis of the cone , cuts all the generators of the cone.
• An ellipse is the set of all points in a plane for which the sum of the distances from the two fixed points (the foci) in the plane is constant
• An ellipse is also defined  as a curve traced by a point, moving in a plane such that the sum of its distances from two fixed points is always the same.

Construction of Ellipse

1. When the  distance of the directrix from the focus and eccentricity is given.
2. Major axis and minor axis is given.
3. Arc of circle method
4. Concetric circle method
5. Oblong method
6. Loop of the thread method

 

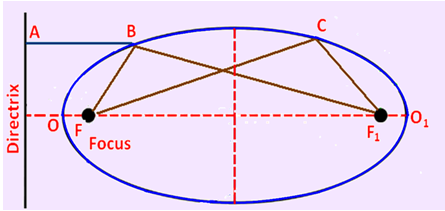
Figure 4. illustrating an ellipse.

Focus-Directrix or Eccentricity Method
Given : the distance of focus from the directrix and  eccentricity
Figure 5. shows the method of drawing an ellipse if the distance of focus from the directrix is 80 mm and the eccentricity is 3/4.

1. Draw the directrix AB and axis CC’
2. Mark F on CC’ such that CF = 80 mm.
3. Divide CF into 7 equal parts and mark V at the fourth division from C. Now, e = FV/ CV = 3/4.
4. At V, erect a perpendicular VB = VF. Join CB. Through F, draw a line at 45° to meet CB produced at D. Through D, drop a perpendicular DV’ on CC’. Mark O at the midpoint of V– V’.