Lecture 8
Properties of plane surfaces I: First moment and centroid of area

Having deal with trusses and frictional forces, we now change gears and go on to discuss some properties of surfaces mathematically. Of course we keep connecting these concepts to physical situations.

The first thing that we discuss is the properties of surfaces. This is motivated by the fact is general the forces do not act at a single point but are distributes over a body. For example the gravitational force pulling an object down acts over the entire object. Similarly a plate immersed in water, for example has the pressure acting on it over the entire surface. Thus we would like to know at which point does the force effectively act? For example in the case of an object in a gravitational field, it is the centre of gravity where the force acts effectively. In this lecture we develop important mathematical concepts to deal with such forces. Let us start with the first moment of an area and the centroid .

First moment of an area and the centroid: We first consider an area in a plane; let us call it the X-Y plane (see figure 1).

The first moment M_X of the area about the x-axis is defined as follows. Take small area element of area ΔA and multiply it by its y-coordinate, i.e. its perpendicular distance from the X-axis, and then sum over the entire area; the sum obviously goes over to an integral in the continuous limit. Thus

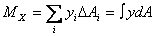
Similarly the first moment M_Y of the area about the y-axis is defined by multiplying the elemental area ΔA by its x-coordinate, i.e. its perpendicular distance from the Y-axis, and summing or integrating it over the entire area. Thus

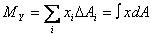
This is shown in figure 2.