Let us now solve a couple of simple standard
examples involving static friction/kinetic friction.
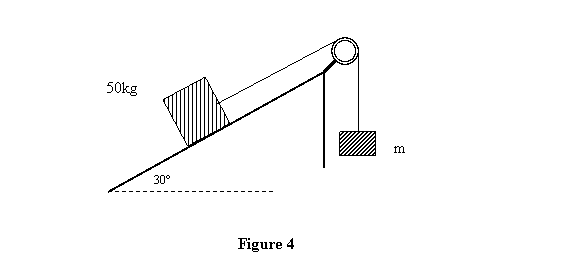
Example 1: A 50kg block
is on an inclined plane of 30°. The coefficient of
static friction between the block and the plane is 0.3.
We wish to determine the range of m under the block
will be in equilibrium (see figure 4).
The very first question that we address
first is: why is there a range of m ? It is
because of the friction. If friction were absent, there
is only one value of m ,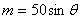 
,
that will balance the 50kg mass. On the other
hand, friction can adjust itself according to the kind
of motion; it can even change direction depending upon
which way is the 50kg block slipping. Thus
when friction is present, there is range of m ,
starting from when the 50kg block has a tendency
to slide down the ramp to when m pulls it up
the ramp. Let us calculate these values. We first take
the case when the 50kg block is about to slide
down the ramp. At that point, the friction will be pointing
up with its magnitude at its maximum value. In that case
the free body diagram of 50kg block is as follows.
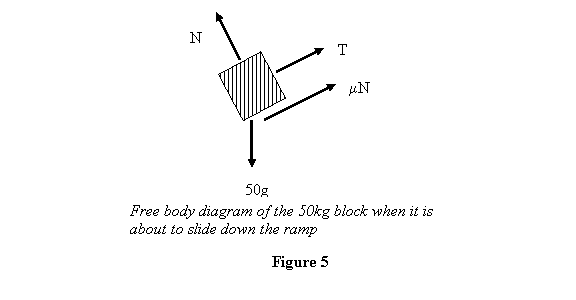
A reminder here that a free body diagram
is the one where we isolate a body and replace all other
elements in contact with it by the forces they apply
on the body. Thus the ramp surface is replaced by its
normal reaction N,
and the frictional force (max) µN, and
the string attached with m is replaced by the
corresponding tension T in it. We reiterate
that we have taken the direction of up the ramp because
we have assumed the block to be sliding down and we have
taken the friction at its maximum possible value. This
gives us the smallest possible mass m .
Taking directions along the ramp to be
the x-direction and that perpendicular to it to be the
y-direction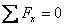 
gives
50g sin30 = T + µ N
Similarly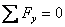 
gives
N = 50g sin30
To get T, we apply the force balance equation to mass m to
get
T = mg
Solving these equations with g = 10ms -2 gives
m = 7.68kg.
Now as we start increasing m,
the frictional force would become smaller and smaller
than its maximum value µN , eventually changing
direction and increasing up to in the µN in
the opposite direction. The free body diagram of 50
kg block
will then look like (note that the direction of friction
is opposite to that in figure 5) figure 6.
Then 
(taking
the x-axis along the ramp) implies that
T = 50g sin30 + µN
The other two equations remain the same as in the previous
case. Solution of these equations gives
m = 42.32kg
Thus we see that due to friction, there is a range of
mass m from 7.68 kg to 42.32 kg that
can balance the 50 kg weight on the ramp; for
all the values of m between the values determined above,
the frictional force will be less than its maximum value.
I leave this example by asking you: at what value of m will
the frictional force be zero?
We now take certain specific example of friction viz.
rotation of a solid cylinder against a dry surface; this
is known as dry thrust waving. We then discuss the case
of belt friction and finally the square screw thread
and the screw jack. In these discussions we closely follow
the book by Shames on Engineering Mechanics.
Example 2: Let us first
take the case of a cylinder of radiation R and
mass m kept
vertically on a rough surface. It is to be rotated about
the vertical by applying a torque T. We wish
to calculate T when the cylinder is about to
rotate. Or in other words what is value of maximum T so
that the cylinder does not rotate see figure 7). The
coefficient of friction between the cylinder and the
surface is µ.
In this example, we will have to consider the torque generated
by friction. To do this, let us consider a ring of radius
r and thickness 'dr' and see how much frictional
force does this experience (see figure 8)?
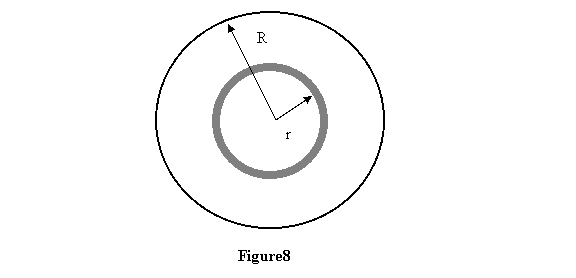
If we assume the normal reaction to be evenly distributed,
then normal reaction on the ring is
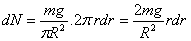
The frictional force on it at radius r is therefore
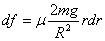
And the torque due to this friction is therefore
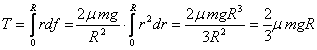
That is the maximum torque that can be tolerated against
friction. Of course we have assumed in this derivation
that the weight of the cylinder is evenly distributed.
If the weight is concentrated more towards the centre, T would
be less and it would be more if the weight is more towards
the periphery. The next example that we take is that
of a block on a ramp again.
Example 3: A block of
mass 100 kg is
on a ramp of angle 30°. We wish to determine
the magnitude and direction of the frictional
force for the applied force F = 600N, F = 500N and F
= 100N .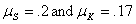 
This
is a problem where we do not know a priori whether the
block will be moving up or down the plane or whether
it will have a tendency to move up or down the plane.
So while solving we have to keep it mind :
- Whether the block will remain stationary or move.
- Which way does the block have tendency to move or
which way does it move?
That will determine the direction of friction.
To get the answer, we see that if the
maximum possible static friction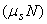 
is
not able to stop the block, it will move and in that
case the friction will be kinetic. We will check that
as we solve the problem. To understand which way will
be the friction act, let us first assume that there is
no friction and calculate the corresponding Fo for
equilibrium. If applied F is greater Fo than
the block will have a tendency to move up, otherwise
it will have a tendency to move down the plane. Free-body
diagram of the block looks (friction = 0) as follows.
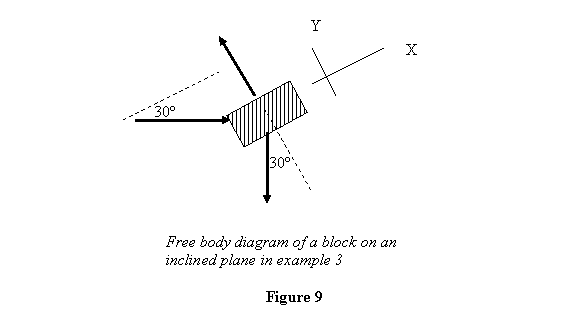
Taking x-axis along the plane and y-axis perpendicular
to it (see figure) we get from the equilibrium conditions
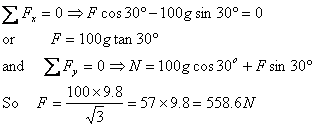
Let us see what happens if we increase F beyond 558.6N.
In that case the component of F up the slope
will increase and the block will have a tendency to move
up. Let us now answer the second question whether at 600N
( Fcos30° - 100gsin
30° )> Max.
Friction OR ( Fcos30° - 100gsin θ ) < Max
Friction
From
N=100gcos30° +Fsin30°
we get
N=981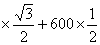 
=490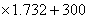 
=848.68+300=1148.68N
Thus maximum value of frictional force is
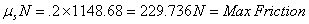
On the other hand,
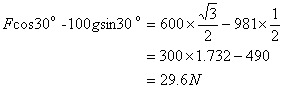
Thus only 29.6N of frictional force is required
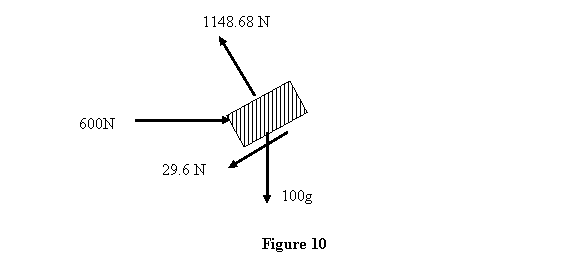
to keep the block in equilibrium. This is well below
the maximum possible frictional force. So under 600N, the
block will be in equilibrium and the direction of friction
will be down the plane. The free body diagram will look
as follows in this case.
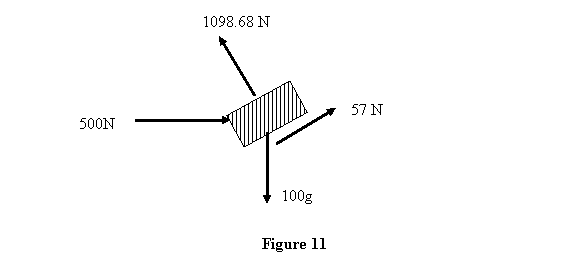
Now we consider the case when the horizontal
push is changed to 500N.
At F = 500N ( Fcos30° - 100gsin30°)
will be negative so the block will have a tendency
to slide down. Let us again calculate the maximum
possible frictional force µsN and ( 100gsin30º -
Fcos30º ) for F = 500N .
N= 100gcos30° -
Fsin30°
=848.68+250=1098.68
and
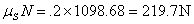
Further
100gsin30° -Fcos30° =490 -
250 × 1.732
=57N
which is again well below the maximum
frictional force of 219.7N. So the block will remain
in equilibrium with its free-body diagram a follows.
Next we consider the case of the force
pushing the block to be 100N. At F = 100N obviously
the block as a tendency to slide down since it does so
for F = 500N.
For 100N case
N = 100gcos30° +Fsin30°
=848.68+50
=898.68 N
and
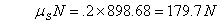
Further
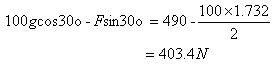
This force is larger than the maximum
frictional (static) force. Thus the block will
start sliding down, However when the block slides down,
the friction will no more be given by µsN
but by µkN.
Thus the frictional force is = .17x 898.68N = 152.8N.
Thus the free body diagram of the block will look as
given below.
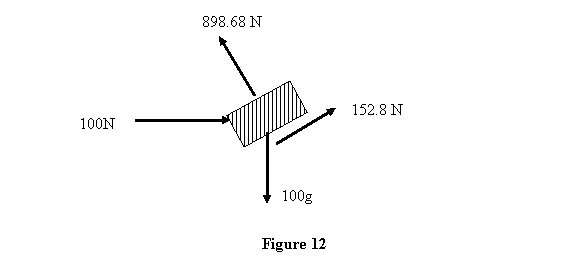
Another way of doing this problem would be to apply the
external force F and calculate the
frictional force required to keep the body in
equilibrium by assuming a direction for the frictional
force. This would give both the direction and
magnitude of the frictional force required for
equilibrium of the block. If this force is below µsN,
the body will remain in equilibrium; if it exceeds µsN, it will move.
Next we consider friction on a belt or
a rope going around an object. For simplicity, we consider
the rope to be going around a pulley and making contact
with it over an angle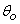 
.Let T1 >T2 so
that the rope has a tendency to slide to the right and
therefore experiences a frictional force to the left.
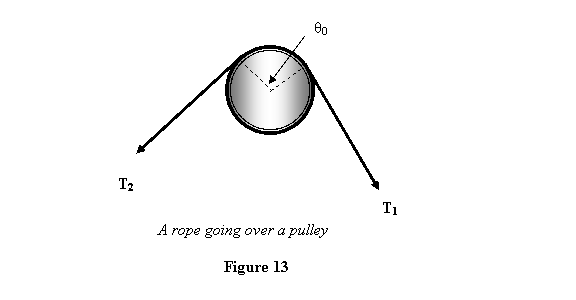
Let us now consider a small section of
angular width 
so
and see how it gets balanced. Let the normal to this
section be shown by the dotted line.
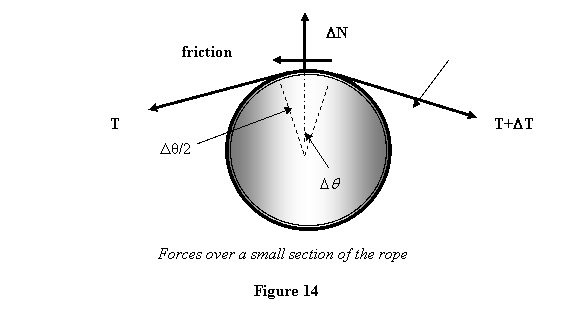
Normal
reaction in this direction balances the components
of T and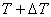 
in
the opposite direction so that.
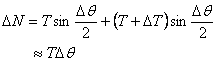
The frictional force is balanced by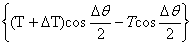 
so
that
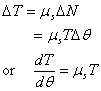
Solving this equation, we get
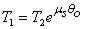
Thus tension in the rope increases exponentially
with the contact angle. Notice that the relationship T1
=T2 does not depend on the radius of
the pulley but only on the contact angle. Thus, even
if the shape of the contact is not perfectly circular,
the same derivation applies and the relationship between
T1 and T2 remains the same, depending only
on the contact angle.
Because of the relationship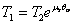 
,
if we take a cylindrical object - say a pencil -and let
a string pass over it, then 
.
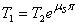If we wrap the string once more, then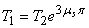 
,
and so on. This is nicely demonstrated by balancing a
heavy object like a bottle filled with water by a light
object like a bunch of keys tied on two ends of a string
and the string itself going many times over a rough stick.
More you wrap the string, a bigger filled bottle would
you be able to balance with the same bunch of keys. If
you measure the weights of the objects carefully, you
should be able to check the relationship derived above
and also get the value of the coefficient of friction
(see figure 15).
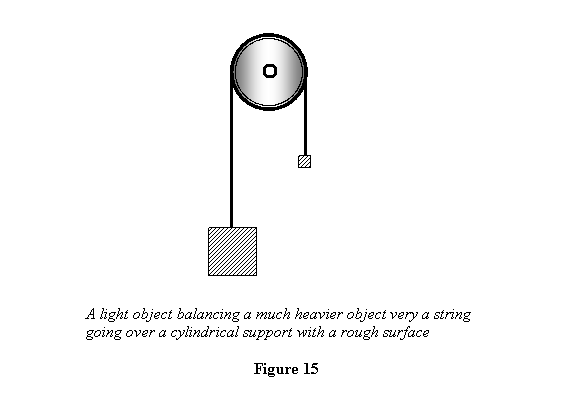
We unknowingly use the effect discussed above when we
wrap a clothes-string over the nail on which it is tied
many times to make it tight. Similarly boats are tied
with the ropes holding them going over a pole many times.
We now solve an example where the surface over which
the rope passes is not cylindrical.
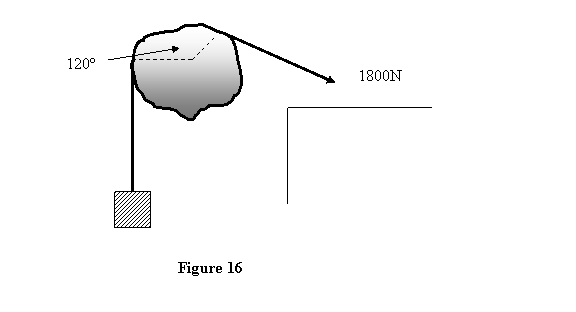
Example: A 70kg load
is being lifted by tying a rope to it and passing the
rope over a tree-trunk. The persons lifting it have to
apply a force of 1800N just when the load starts moving
up. What is the coefficient of static friction between
the tree-trunk and the rope if the contact angle between
the trunk and the rope is 120°? (see figure 16).
Since the rope is in contact with the
rope over an angle of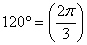 
,
the relationship between T on the slide of the
persons pulling the rope and To on the side
of the load is (friction is towards the load since rope
is about to move towards the persons pulling it)
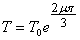
Notice that we are not worrying about
the shape of the tree-trunk but rather only about the
contact angle. It is given that T = 1800N and T0
=70 x 9.8 = 688N .
Therefore we have
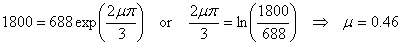
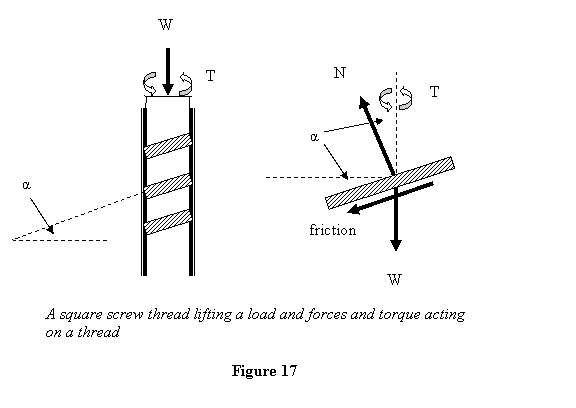
Finally, we take the example of a square screw thread
and a screw jack. In this case a screw with square threads
passes through a nut and we wish to consider the action
of the nut on the screw. You have seen this in a jack
where a load (say a car) is lifted by rotating a screw.
So a load W is lifted by applying a torque T on
the screw (figure 17).
The threads on the screw make an angle α with
the horizontal so that
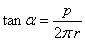
where p is the pitch and r is
the mean radius of the screw. We wish to find the torque T (minimum)
that is needed to lift the load W. When the screw
is being lifted up the nut applies a normal reaction
and a frictional force on the screw shown in figure 16.
Keep in mind that the normal reaction and the friction
act on the periphery of the screw. Thus they also apply
a couple about the vertical axis on the screw. The figure
also shows the load W . Balancing the
force in the vertical direction and the torque
about the vertical axis gives
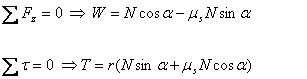
Solving these equations gives
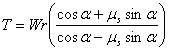
This is the torque needed to lift the load W .
Let us now say that we raise the load and then release
the torque. Will the jack self-lock i.e., would it hold
the load where it is and would not unwind. We want to
find the condition for this. When the screw self-locks,
it will have a tendency to move down. Thus the free body
diagram of the screw in this condition looks as follows.
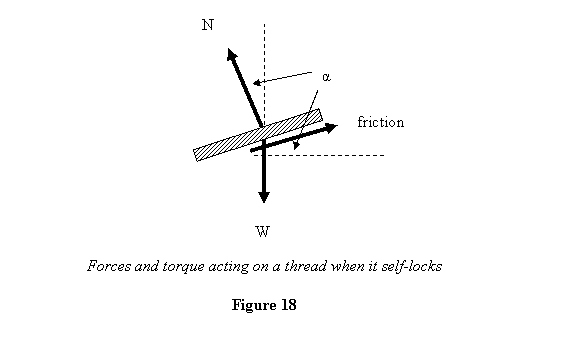
Balancing the vertical force now gives
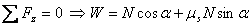
and if the screw is not to unwind, the torque due to the
friction should be large than that due N . Thus
for self-locking we require that
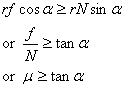
Thus is the condition for self-locking. Under this condition
the screw will not unwind by itself as torque due to
the frictional force would be sufficient to prevent the
unwinding due to the torque arising from N .
Example: Let us now
solve a household example of this. Sometimes
you see that experimental tables or refrigerators at
home have screw-like contraption at their legs to adjust
their heights. Let the radius of such a screw be 2mm.
We wish to know what should be minimum number
of thread per cm so that the screw self-locks for µS =0.15.
For self locking we know that
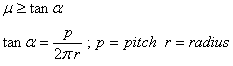
This gives on substitution
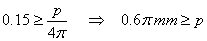
If the number threads per cm is N then
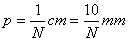
This gives
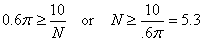
Thus there should be a minimum of 6 threads per centimeter
in order that the screw self-locks itself.
We conclude this lecture with this example.
In this lecture we have learnt about the frictional forces
and how they act under different situations.
