An RC circuit is shown in fig.7.1. Since, in practical circuits,
power is always switched on at certain time, a switch is provided here.
This switch closes at time ![]() .
.
We are interested in finding how voltage across capacitor ![]() changes with
time? We can also assume that voltage across the capacitor is zero
changes with
time? We can also assume that voltage across the capacitor is zero ![]() .
Using Kirchoff's voltage law across the only loop in circuit we can find
the equation relating
.
Using Kirchoff's voltage law across the only loop in circuit we can find
the equation relating ![]() ,
, ![]() and
and ![]() .
.
Using the characterstic equations of capacitors, resistors i.e.,
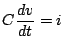 |
|
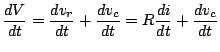 |
|
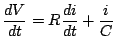 for for |
|
| for |
|
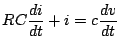 |
|
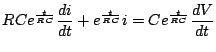 |
|
| For |
|
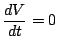 |
|
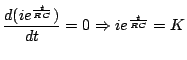 |
|
| Thus, |
Alternatively,
| at |
|
Thus, 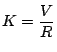 |
| (7.1) |
The curves showing ![]() and
and ![]() are shown in the figures 7.2 and 7.3.
are shown in the figures 7.2 and 7.3.
Consider the figure shown in 7.1. The switch is closed at
![]() .
.
Now,
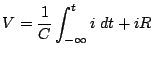 |
|
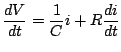 |
|
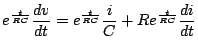 |
|
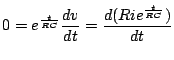 |
|
For RC circuit with source voltage zero, and an initial capacitor
voltage of ![]() , this expression reduces to
, this expression reduces to
![]() .
.
For constant current charging of a capacitor, as shown in 7.4, the analysis:
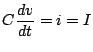 |
(7.2) |
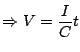 |
(7.3) |
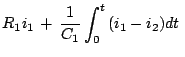 |
|||
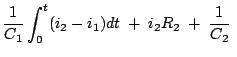 |
|||
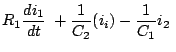 |
|||
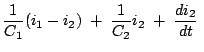 |
|||
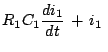 |
|||
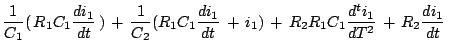 |
0 | ||
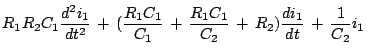 |
The switch is turned off at ![]() sec. There is no charge on the capacitor initially.
Therefore, after
sec. There is no charge on the capacitor initially.
Therefore, after ![]() and before
and before ![]() , the circuit is equivalent to figure 7.7
, the circuit is equivalent to figure 7.7
Taking thevenin equivalent in the direction of the arrow leads to figure 7.8
Therefore ,
After
![]() , the switch is once again thrown open and the equivalent circuit is shown in figure 7.9
, the switch is once again thrown open and the equivalent circuit is shown in figure 7.9
Now,
The graph of ![]() with time is shown in figure 7.10
with time is shown in figure 7.10