4.1.3 Dielectric Displacement and Susceptibility
Consider a vacuum plate capacitor configuration as shown below:
| Figure 4.3 Parallel plate vacuum capacitor |
| Figure 4.4 Parallel plate capacitor with a dielectric |
For a vacuum capacitor
OR
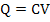 |
(4.7) |
where capacitance of the vacuum is given as Cvac = (ε0A)(d) where εo is the permittivity of free space and is equal to 8.85x10-12 F/m.
If one inserts a dielectric between plates, then capacitance gets modified as
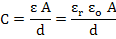 |
(4.8) |
Where εr is dielectric permittivity or more commonly (but not accurately) as relative dielectric constant with value greater than 1.
OR
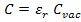 |
(4.9) |
(4.9)
Thus, inserting a dielectric leads to an increase in the stored charge in the capacitor as shown below.
Basically, εr signified some sort of interaction between the material and electromagnetic field.
Figure 4.5 Q-V plot with effect of εr |
Now the question is: Why does inserting a dielectric increases the capacitance of a capacitor?
We will see this in the following sections. |