|
(contd from previous...) Concept of Flow Potential and Flow Resistance
The velocity V in the above equation is usually substituted in terms of flow rate Q , since, under steady state, the flow rate remains constant throughout the pipe even if its diameter changes. Therefore, replacing V in Eq. (35.11) as 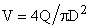 we finally get we finally get
In a situation where f becomes independent of Re, the flow resistance expressed by Eg. (35.13) becomes simply a function of the pipe geometry. With the help of Eq. (35.10), Eq. (35.12) can be written as
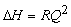 |
(35.14) |
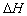 in Eq. (35.14) is the head causing the flow and is defined as the difference in flow potentials between A and B. in Eq. (35.14) is the head causing the flow and is defined as the difference in flow potentials between A and B.
This equation is comparable to the voltage-current relationship in a purely resistive electrical circuit. In a purely resistive electrical circuit, 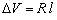 , where , where 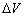 is the voltage or electrical potential difference across a resistor whose resistance is R and the electrical current flowing through it is I. is the voltage or electrical potential difference across a resistor whose resistance is R and the electrical current flowing through it is I.
- The difference however is that while the voltage drop in an electrical circuit is linearly proportional to current, the difference in the flow potential in a fluid circuit is proportional to the square of the flow rate.
- Therefore, the fluid flow system as shown in Fig. 35.3 and described by Eq. (35.14) can be expressed by an equivalent electrical network system as shown in Fig. 35.4.
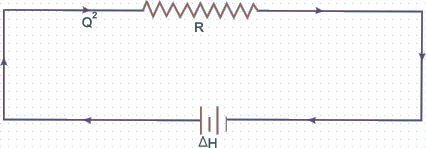
Fig 35.4 Equivalent electrical network system for a simple pipe flow problem shown in Fig.35.3
|