Compressor characteristics
The theoretical and actual head-discharge relationships of a centrifugal compressor are same as those of a centrifugal pump as described in Module 1. However, the performance of a compressor is usually specified by curves of delivery pressure and temperature against mass flow rate for various fixed values of rotational speed at given values of inlet pressure and temperature. It is always advisable to plot such performance characteristic curves with dimensionless variables. To find these dimensionless variables, we start with a implicit functional relationship of all the variables as
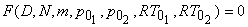 |
(8.1) |
where D = characteristic linear dimension of the machine, N = rotational, m = mass flow rate,
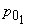 = stagnation pressure at compressor inlet, = stagnation pressure at compressor inlet,
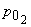 = stagnation pressure at compressor outlet, = stagnation pressure at compressor outlet,
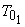 = stagnation temperature at compressor inlet, = stagnation temperature at compressor inlet,
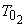 = stagnation temperature at compressor outlet, and R = characteristics gas constant. = stagnation temperature at compressor outlet, and R = characteristics gas constant.
By making use of Buckingham's π theorem, we obtain the non-dimensional groups (π terms) as
The third and fourth non-dimensional groups are defined as 'non-dimensional mass flow' and 'non-dimensional rotational speed' respectively. The physical interpretation of these two non-dimensional groups can be ascertained as follows.
Therefore, the 'non-dimensional mass flow' and 'non-dimensional rotational speed' can be regarded as flow Mach number, 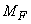 and rotational speed Mach number, and rotational speed Mach number, 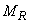 . .
When we are concerned with the performance of a machine of fixed size compressing a specified gas, 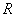 and D may be omitted from the groups and we can write and D may be omitted from the groups and we can write
Function 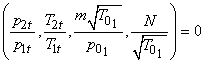 |
|
(8.2) |
Though the terms
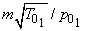 and and
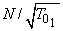 are truly not dimensionless, they are referred as 'non-dimensional mass flow' and 'non-dimensional rotational speed' for practical purpose. The stagnation pressure and temperature ratios are truly not dimensionless, they are referred as 'non-dimensional mass flow' and 'non-dimensional rotational speed' for practical purpose. The stagnation pressure and temperature ratios
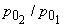 and and
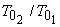 are plotted against are plotted against
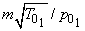 in the form of two families of curves, each curve of a family being drawn for fixed values of in the form of two families of curves, each curve of a family being drawn for fixed values of
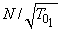 . The two families of curves represent the compressor characteristics. From these curves, it is possible to draw the curves of isentropic efficiency . The two families of curves represent the compressor characteristics. From these curves, it is possible to draw the curves of isentropic efficiency
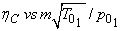 for fixed values of for fixed values of
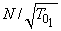 . We can recall, in this context, the definition of the isentropic efficiency as . We can recall, in this context, the definition of the isentropic efficiency as
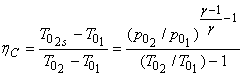 |
(8.3) |
Before describing a typical set of characteristics, it is desirable to consider what might be expected to occur when a valve placed in the delivery line of the compressor running at a constant speed, is slowly opened. When the valve is shut and the mass flow rate is zero, the pressure ratio will have some value. Figure 8.2 indicates a theoretical characteristics curve ABC for a constant speed.
The centrifugal pressure head produced by the action of the impeller on the air trapped between the vanes is represented by the point 'A' in Figure 8.2. As the valve is opened, flow commences and diffuser begins to influence the pressure rise, for which the pressure ratio increases. At some point 'B', efficiency approaches its maximum and the pressure ratio also reaches its maximum. Further increase of mass flow will result in a fall of pressure ratio. For mass flows greatly in excess of that corresponding to the design mass flow, the air angles will be widely different from the vane angles and breakaway of the air will occur. In this hypothetical case, the pressure ratio drops to unity at 'C' , when the valve is fully open and all the power is absorbed in overcoming internal frictional resistances.
In practice, the operating point 'A' could be obtained if desired but a part of the curve between 'A' and 'B' could not be obtained due to surging. It may be explained in the following way. If we suppose that the compressor is operating at a point 'D' on the part of characteristics curve (Figure 8.2) having a positive slope, then a decrease in mass flow will be accompanied by a fall in delivery pressure. If the pressure of the air downstream of the compressor does not fall quickly enough, the air will tend to reverse its direction and will flow back in the direction of the resulting pressure gradient. When this occurs, the pressure ratio drops rapidly causing a further drop in mass flow until the point 'A' is reached, where the mass flow is zero. When the pressure downstream of the compressor has reduced sufficiently due to reduced mass flow rate, the positive flow becomes established again and the compressor picks up to repeat the cycle of events which occurs at high frequency.
This surging of air may not happen immediately when the operating point moves to the left of 'B' because the pressure downstream of the compressor may at first fall at a greater rate than the delivery pressure. As the mass flow is reduced further, the flow reversal may occur and the conditions are unstable between 'A' and 'B'. As long as the operating point is on the part of the characteristics having a negative slope, however, decrease in mass flow is accompanied by a rise in delivery pressure and the operation is stable.
Figure 8.2 The theoretical characteristic curve |
There is an additional limitation to the operating range, between 'B' and 'C'. As the mass flow increases and the pressure decreases, the density is reduced and the radial component of velocity must increase. At constant rotational speed this means an increase in resultant velocity and hence an angle of incidence at the diffuser vane leading edge. At some point say 'E', the position is reached where no further increase in mass flow can be obtained no matter how wide open the control valve is. This point represents the maximum delivery obtainable at the particular rotational speed for which the curve is drawn. This indicates that at some point within the compressor sonic conditions have been reached, causing the limiting maximum mass flow rate to be set as in the case of compressible flow through a converging diverging nozzle. Choking is said to have taken place. Other curves may be obtained for different speeds, so that the actual variation of pressure ratio over the complete range of mass flow and rotational speed will be shown by curves such as those in Figure. 8.3. The left hand extremities of the constant speed curves may be joined up to form surge line, the right hand extremities indicate choking (Figure 8.3).

| Figure 8.3 Variations of pressure ratio over the complete range of mass flow for different rotational speeds |
|