Once the correlation is found, the Fourier transformations are converted back into the physical space. The displacement that yields a maximum in the correlation function over the interrogation area is regarded as the particle displacement. Actually it is not the particle displacement which is computed but the displacement of the interrogation area. The displacement vector is of first order, i.e. the average shift of the particles is geometrically linear within the interrogation window. The size of the interrogation should be suffciently small such that the second order effect, i.e. displacement gradients can be neglected.

Peak detection and displacement estimation

One of the important steps in evaluation of PIV images is to measure the position of correlation peak accurately to sub-pixel accuracy. To increase the accuracy in determining the location of the displacement peak from pixel to sub-pixel accuracy, an analytical function is fitted to the highest correlation peak by using the adjacent correlation values. Various methods of estimating the location of the correlation peak have been proposed. Some of these are peak centroid fit, Gaussian peak fit and the parabolic peak fit. Of the three, the Gaussian fit is most frequently used to estimate the shape of the signal around its peak assuming ideal imaging conditions. This function is

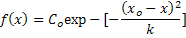
where indicates the exact location of the maximum peak and and k are parametric coeffcients. Using this expression for the main and the adjacent correlation values and the fact that the first derivative of this expression at must be zero, the position can be estimated with sub-pixel accuracy. Generally, a 3-point Gaussian peak fit gives good results. When the particle image size is small, the displacement tends to bias towards integer values. The assumed peak shape does not match the actual shape of the peak and the three point Gaussian estimator cannot represent the true shape of the correlation function. This is called the peak-locking effect. In actual displacement data, the presence of the peak-locking effect can be detected from histogram plot.