Boundary & Initial Conditions

Formulation of the problem requires a complete specification of the geometry of interest and appropriate boundary conditions. An arbitrary domain and bounding surfaces are sketched in Fig. 1.1.

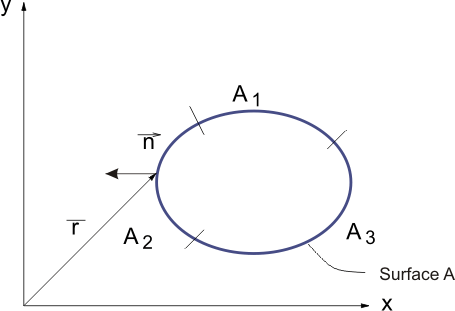
Figure 1.1: Schematic sketch of an arbitrary Domain

The conservation equations are to be applied within the domain. The number of boundary conditions required is generally determined by the order of the highest derivatives appearing in each independent variable in the governing differential equations.

The unsteady problems governed by a first derivative in time will require initial condition in order to carry out the time integration. The diffusion terms require two spatial boundary conditions for each coordinate in which a second derivative appears.