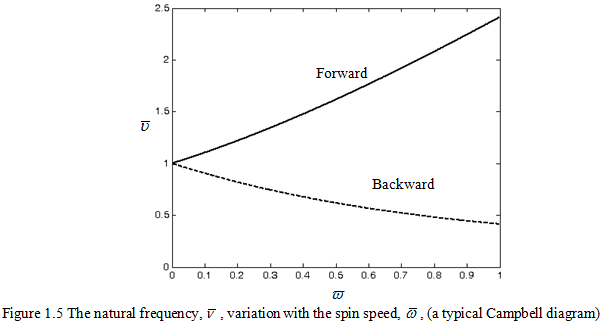
In order to calculate the critical speeds of cylindrical shafts with several discs and bearings the general theory of Reynolds (Dunkerley, 1895) was applied. The gyroscopic effect was also considered, together with its dependence on speed (i.e., a Campbell diagram see Fig. 1.5) . Dunkerley found through numerous measurements, the relationship known today by that of Southwell, by which the first critical speed can be calculated, even for multi-degree-of-freedom rotor cases. The first sentence of Dunkerley’s paper reads, "It is well known that every shaft, however nearly balanced, when driven at a particular speed, bends, and, unless the amount of deflection be limited, might even break, although at higher speeds the shaft again runs true. This particular speed or critical speed depends on the manner in which the shaft is supported, its size and modulus of elasticity, and the sizes, weights, and positions of any pulleys it carries.” This was the first use of the term critical speed for the resonance rotational speed.
Even with the general knowledge of critical speeds, the shaft behaviour at any general speed was still unclear but more was learnt from the calculation of unbalance vibrations, as given by Föppl (1895).

He used an undamped model to show that an unbalanced disc would whirl synchronously with the heavy side (shown as black spot) flying out (Fig. 1.4a) when the rotation was subcritical and with the heavy side flying in (Fig. 1.4b) when the rotation was supercritical. Also the behaviour of Laval rotors at high speed was confirmed by his theory.

 

It was unfortunate that engineers of those days were under a confusion of concepts by equating the Rankine's whirling speed with the Dunkerley's critical speed. This was particularly frustating since Rankine was far more well known than Dunkerley and, as a result, his predictions were widely accepted and became responsible for discouraging the development of high speed rotors for almost 50 years. It was in England in 1916 that things came to the end. Kerr published experimental evidence that a second critical speed existed, and it was obvious to all that a second critical speed could only be attained by the safe traversal of the first critical speed.

The first recorded fundamental theory of rotor dynamics can be found in a classic paper of Jeffcott in 1919. Jeffcott confirmed Föppl's prediction that a stable supercritical solution existed and he extended Foppl's analysis by including external damping (i.e., damping forces that depend upon only the absolute velocity of the rotor, whereas the internal damping comes from rate of deformation of the shaft) and showed that the phase of the heavy spot varies continuously as the rotation rate passes through the critical speed. We can appreciate Jeffcott’s great contributions if we recall that a flexible shaft of negligible mass with a rigid disc at the midspan is called a Jeffcott rotor (Fig. 1.3b). The bearings are rigidly supported, and viscous damping acts to oppose absolute motion of the disc. This simplified model is also called the Laval rotor, named after de Laval.