1.5 Atomic Orbitals
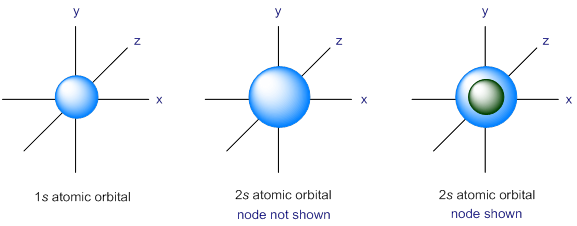
An orbital is a three-dimensional region around the nucleus where the probability of finding an electron is high. Mathematical calculations and experimental evidence indicate that the s atomic orbital is a sphere with the nucleus at its center. According to the Heisenberg uncertainty principle, both the location and the momentum of an atomic particle cannot be determined simultaneously.
We can never say exactly where an electron is. We can only describe its probable location. If we say that an electron occupies a 1s atomic orbital, it means, there is a greater than 90% probability of finding the electron in the space which is defined as the sphere for s orbital. The average distance from the nucleus is greater for an electron in a 2s atomic orbital than for an electron in a 1s atomic orbital. Consequently, the average electron density in a 2s atomic orbital is less than the average electron density in a 1s atomic orbital. An electron in a 1s atomic orbital can be anywhere within the 1s sphere, but there is a node in a 2s atomic orbital where the probability of finding an electron is zero.
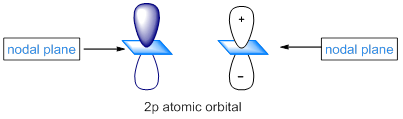
Unlike s atomic orbitals, p atomic orbitals have two lobes which are of opposite phase. These two phases can be designated by plus and minus signs.
A plane that passes through the center of the nucleus of the p atomic orbital is called, nodal plane that bisects the two lobes. There is zero probability of finding an electron in the nodal plane of the p orbital.
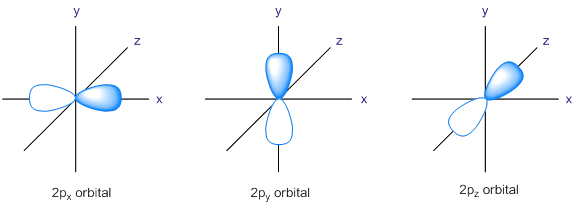
We have seen that there are three degenerate p atomic orbitals. The px orbital is symmetrical about the x -axis, py the orbital is symmetrical about the y -axis, and pz the orbital is symmetrical about the z -axis. All these p orbitals are perpendicular to each other. The energy of a 2p atomic orbital is slightly greater than that of a 2s atomic orbital.