Similarly the boundary conditions can be simplified to
Integration of equation (19.4) yields
In which we put the boundary conditions as in equation 19.3a and 19.4
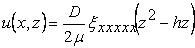 |
(19.6) |
Substitution of 19.6 in equation 19.2 followed by integration gives rise to the following expression for vertical displacement of the cover plate in the region 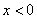 where it is attached with the adhesive film where it is attached with the adhesive film
Using boundary condition 19.2 and noting that 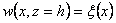 , we obtain, , we obtain,
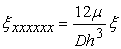 for for 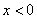 |
(19.7) |
And in the region 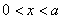 , where the film is not in contact with film, , where the film is not in contact with film,
 for for 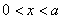 |
(19.8) |
Equations 19.7 and 19.8 together demand ten constants which are to be determined using ten boundary conditions:
Since the deformation of the flexible plate film must vanish far away from the contact line,
Continuity of the displacement, slope, bending moment, vertical shear force and the pressure at the contact line results in
Finally, at 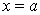 , where the flexible plate is freely pivoted while being lifted vertically by an amount , where the flexible plate is freely pivoted while being lifted vertically by an amount  , the boundary conditions are , the boundary conditions are
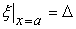 , , 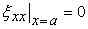
|
(19.11) |
Thus equations 19.9 to 19.11 present nine boundary conditions. One additional boundary condition is obtained by considering that at the line of contact between the flexible plate and film the stress concentration is maximized, which implies that
1 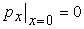
|
(19.12) |
|