|
It should be noted that the virtual displacement 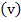 is any arbitrary displacement. It can be chosen to vary on macroscopic or microscopic level. If we choose is any arbitrary displacement. It can be chosen to vary on macroscopic or microscopic level. If we choose 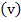 to vary only on macroscopic level and be a constant on microscopic level then we get the macroscopic equilibrium equation as to vary only on macroscopic level and be a constant on microscopic level then we get the macroscopic equilibrium equation as
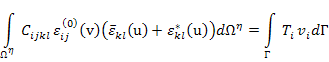 |
(7.137) |
Here, 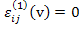 for the chosen variation of virtual displacement. However, if we choose for the chosen variation of virtual displacement. However, if we choose 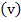 to vary only on microscopic level and be a constant on macroscopic level then we get the microscopic equilibrium equation as to vary only on microscopic level and be a constant on macroscopic level then we get the microscopic equilibrium equation as
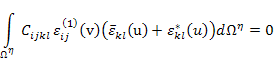 |
(7.138) |
Here, 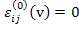 for the chosen variation of virtual displacement. Since for the chosen variation of virtual displacement. Since 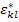 varies periodically, Equation (7.137) and Equation (7.138) may be simplified assuming varies periodically, Equation (7.137) and Equation (7.138) may be simplified assuming 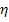 approaching zero in the limit as approaching zero in the limit as
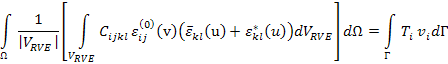 |
(7.139) |
and
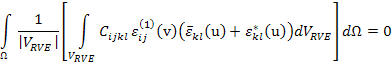 |
(7.140) |
Equation (7.140) to be true, the integration term over the RVE should be zero. This leads to the following condition:
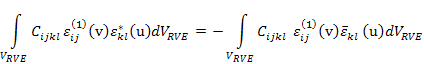 |
(7.141) |
Here, in general, the strain 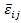 is not known. However, for a linear problem, any arbitrary is not known. However, for a linear problem, any arbitrary 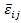 can be written as linear combination of unit strains as given in the following equation. The unit strains in the following equation are given for 3D case. can be written as linear combination of unit strains as given in the following equation. The unit strains in the following equation are given for 3D case.
|