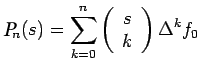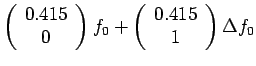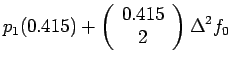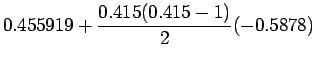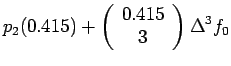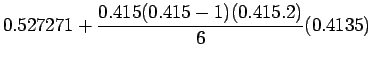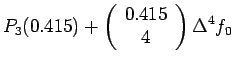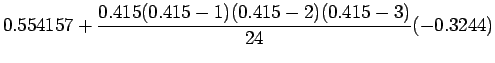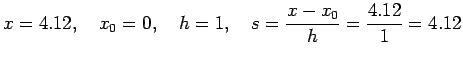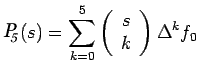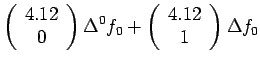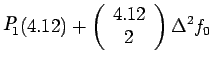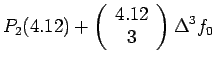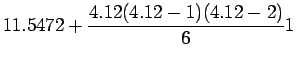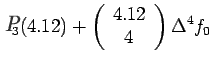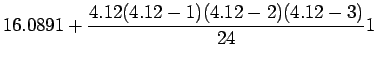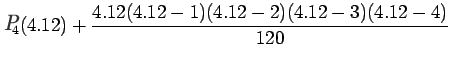where
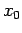
is the starting point (sometimes, for convenience,
the middle data point is taken as
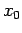
and in such a case the
integer
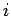
is allowed to take both negative and positive values.)
and
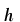
is the step size. Further it is enough to calculate simple
differences rather than the divided differences as in the
non-uniformly placed data set case. These simple differences can
be forward difference
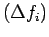
or back differences
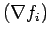
. We will first look at forward differences and
the interpolation polynomial based on
forward differences.
The first order forward difference
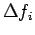
is defined as
Since we already know Newton interpolation polynomial in terms of
divided differences, to derive or generate Newton interpolation
polynomial in terms of forward differences it is enough to express
forward differences in terms of divided differences.
Recall the definition of first divided difference
![$ f[x_{0},x_{1}]$](img212.png)
,
This is known as Gregory-Newton forward difference interpolation
polynomial. For Convenience while constructing (10) one can first
generate a forward difference table and use the
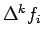
from the table. Suppose we have data set
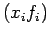
,
then forward difference table looks as follows:
![]() correspond to a sequence
correspond to a sequence ![]() of
equally spaced points. Here we can assume that
of
equally spaced points. Here we can assume that
![$\displaystyle f[x_{0},x_{1}]=\frac{f(x_{1})-f(x_{0})}{x_{1}-x_{0}}=\frac{f_{1}-f_{0}}{h}=\frac{\Delta
f_{0}}{h}$](img213.png)
![% latex2html id marker 2701
$\displaystyle \therefore f[x_{i},x_{i+1},...x_{i+k}]=\frac{\Delta^{k}f_{i}}{k!h^{k}}$](img224.png)
![$\displaystyle f[x_{0},x_{1}...x_{k}]=\frac{\Delta^{k}f_{0}}{k!h^{k}}$](img226.png)
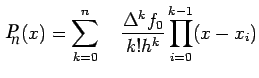
![]()
![]()
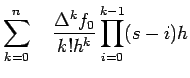
![$\displaystyle \sum\limits_{k=0}^{n}\quad\frac{\Delta^{k}f_{0}}{k!h^{k}}\quad[s(s-1).......(s-k+1)]h^{k}$](img233.png)
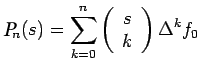
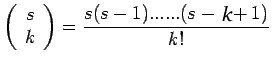
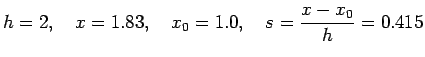
![]() Newton Gregory forward difference interpolation
polynomial is given by:
Newton Gregory forward difference interpolation
polynomial is given by: