Lecture 24
Harmonic oscillator I: Introduction

Having analyzed the motion of particles in different situations, let us now focus on a very special kind of motion: that of oscillations. This is a very general kind of motion seen around you: A partial moving around the bottom of a cup, a pendulum swinging, a clamped rod vibrating about its equilibrium position or a string vibrating. A good first approximation to these motions is the simple harmonic oscillation. Let us see what does that mean? At a stable equilibrium point, the force on a body is zero; not only that, as a particle moves away from equilibrium, its potential energy increases and it is pulled back towards the equilibrium point. Thus around a stable equilibrium point x₀ (for simplicity, let me take one-dimensional motion) the potential energy 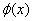  can be written as

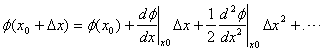
Since at an equilibrium point, the force F(x₀ ) on the particle vanishes,

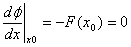
Further, because Φ(x) has a minimum at x₀ , this gives

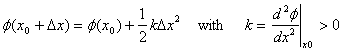
Writing 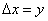  I get

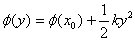
and the corresponding equation of motion for a mass m as

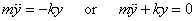
As I will show a little later, the solution of this equation is of the form

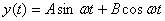
and is known as the simple harmonic motion. It is the simplest possible motion about a stable equilibrium point. Of course if k = 0 , the force will have higher order dependence on y and the motion becomes more complicated. Further, even if 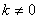, if we include higher order terms, the resulting motion will become more complex. It is for this reason that we call the motion above simple harmonic motion. We will see that this itself is quite a rich system. A system that performs simple harmonic motion is called a simple harmonic oscillator. A prototype if this system is the spring-mass system with k being the spring constant and m the mass of the block on the spring (figure 1).

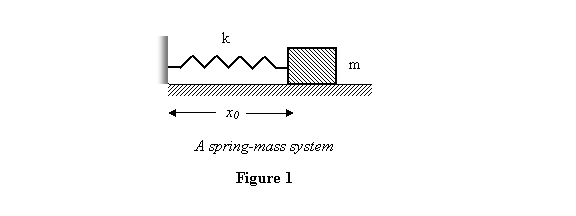
In these lectures, I will talk about the motion of this system and how it is represented by a phasor diagram. I will then introduce damping into the system. The simplest damping is a constant opposing force like friction and next level is a damping proportional to the velocity. Then I will apply a force on the system and see the motion of force damped and undamped oscillator. Along the way, I will solve many examples to show wide applicability of simple harmonic motion.

To start with let us take our prototype system of mass and spring with unstretched length of the spring 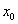so that equilibrium distance of the mass is 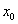. Now when the mass is displaced about 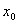by x in the positive direction, the force is in negative direction so that

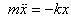
or

This is the general equation for simple harmonic oscillator. Recall that in such cases we assume a solution of the form

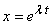
and substitute it in the equation to get

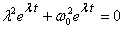
Since this equation is true for all times, we should have

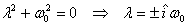
Thus there are two solution 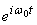and 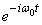. A general solution is then given in terms of a linear combination of the two solutions so let us write

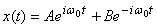
Since 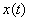is real it is clear that 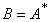. Thus

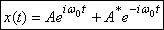
If we take A = A_R + iA_I , where both A_R   and A_I   are real then the solution above takes the form

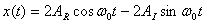
which alternatively can be written as

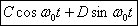
Another equivalent way of writing the solution is

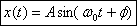or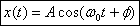

where

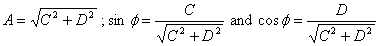
A is the maximum distance that the mass travels during a simple harmonic oscillation. It is known as the amplitude of oscillation. The quantity 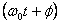is known as the phase with Φ being the initial phase. All the boxed equations above are equivalent ways of writing the solution for a harmonic oscillator. The general graph depicting the solution 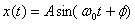is given in figure 2.

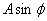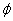Thus A is the maximum distance traveled by the block and gives its initial displacement. The constants C and D or A and are determined by the initial conditions, i.e. initial displacement and velocity of the mass. In general any two conditions are enough to determine the constants.

For a displacement

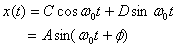
the velocity of the mass is given by

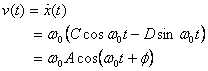
Thus the maximum possible magnitude of the velocity is ω₀ A . The general displacement and the corresponding velocity of the mass with respect to time are displayed in figure 3.

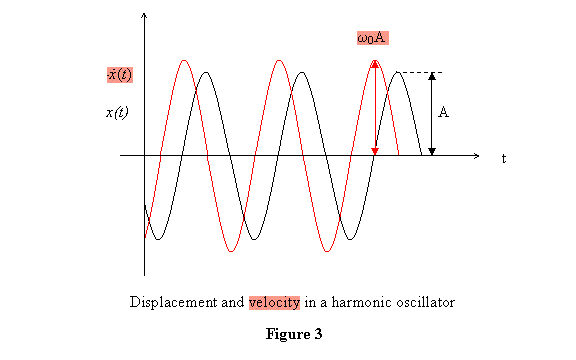
It is clear from the figure that for a given displacement, the velocity is such that when displacement is at its maximum or minimum, the velocity is zero and when the displacement is zero, the velocity has the largest magnitude. This is physically clear. When the spring is compressed or stretched to its maximum, the particle is at rest and when the particle passes through the equilibrium point, its speed is at its maximum. Let me now solve a few examples.