Karhunen-Loeve Transform (KLT ) (continued)
Since the KTL is unitary,
We may now write
which form eqn  , |
we get |
 |
As 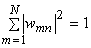 , |
we have |
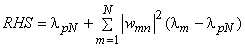 |
ie |
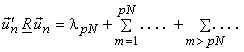 |
which is |
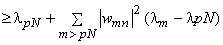 |
(Since 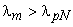 for for  ) )
Thus from eqn for |
 |
we have |
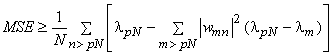 |
The second summation |
ie  |
and since |
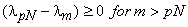 |
we have |
 |
ie 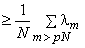 |
which is = MSE for KTL |
This concludes that the MSE for any other linear transform
 exceeds that of the KTL transform. exceeds that of the KTL transform. |