Flow Past a Source

When a uniform flow is added to that due to a source -

• fluid emitted from the source is swept away in the downstream direction
• stream function and velocity potential for this flow will be the sum of those for uniform flow and source

Stream function;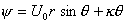     

Velocity Potential;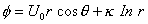  

So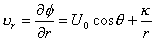                           

and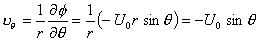                          

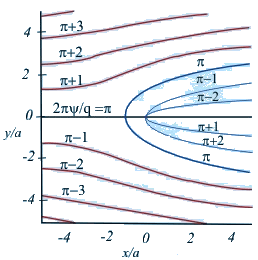
Fig 23.1  The streamlines of the flow past a line source for equal increments of 2πψ/q
The Plane coordinates are x/a, y/a where a=k/u

Explanation of Figure

• At the point x = -a, y = 0 fluid velocity is zero.
• This is called stagnation point of the flow
• Here the source flow is turned around by the oncoming uniform flow
• The parabolic streamline passing through stagnation point seperates uniform flow from the source flow.
• The streamline becomes parallel to x axis as

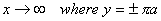
Flow Past Vortex

when uniform flow is superimposed with a vortex flow -

• Flow will be asymmetrical about x - axis
• Again stream function and velocity potential will be the sum of those for uniform flow and vortex flow

Stream Function: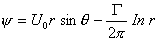   

Velocity Potential: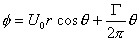   

so that;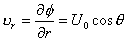
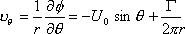