|
Ideal
Fluid
- Consider a hypothetical fluid having
a zero viscosity ( μ = 0). Such a fluid is called an
ideal fluid
and the resulting motion is called as ideal or
inviscid flow. In an ideal flow, there is no existence
of shear force because of vanishing viscosity.
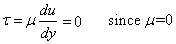
- All the fluids in reality
have viscosity (μ > 0) and hence they are termed as real fluid and their
motion is known as viscous flow.
- Under certain situations of very
high velocity flow of viscous fluids, an accurate analysis
of flow field away from a solid surface can be made from
the ideal flow theory.
Non-Newtonian Fluids
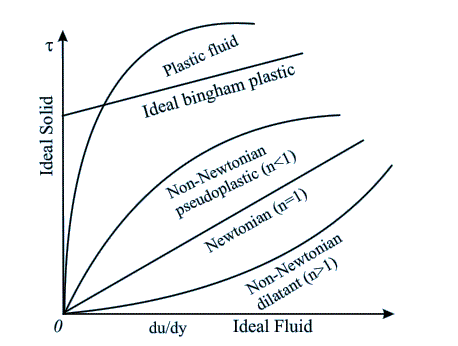
Figure 2.1
Shear stress and deformation rate relationship of different
fluids
- The abscissa in Fig. 2.1 represents the
behaviour of ideal fluids since for the ideal fluids the
resistance to shearing deformation rate is always zero,
and hence they exhibit zero shear stress under any condition
of flow.
- The ordinate represents the ideal solid
for there is no deformation rate under any loading condition.
- The Newtonian fluids behave
according to the law that shear stress is linearly
proportional to velocity gradient or rate of shear strain
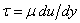 . Thus for these fluids, the plot of
shear stress against velocity gradient is a straight line
through the origin. The slope of the line determines the
viscosity. . Thus for these fluids, the plot of
shear stress against velocity gradient is a straight line
through the origin. The slope of the line determines the
viscosity.
- The non-Newtonian fluids are further classified as pseudo-plastic, dilatant
and Bingham
plastic.
|