Similarity
and Dimensional Analysis
or, with another arrangement of the π
terms,
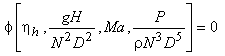 |
(3.2) |
If data obtained from tests on model machine, are plotted so as to show the variation of dimensionless parameters 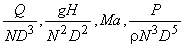 with one another, then the graphs are applicable to any machine in the same homologous series. The curves for other homologous series would naturally be different. with one another, then the graphs are applicable to any machine in the same homologous series. The curves for other homologous series would naturally be different.
Specific
Speed
The performance or operating conditions for a
turbine handling a particular fluid are usually
expressed by the values of N , P and H ,
and for a pump by N , Q and H .
It is important to know the range of these operating
parameters covered by a machine of a particular
shape (homologous series) at high efficiency. Such information enables
us to select the type of machine best suited
to a particular application, and thus serves
as a starting point in its design. Therefore
a parameter independent of the size of the machine D is
required which will be the characteristic of
all the machines of a homologous series. A parameter
involving N , P and H but
not D is obtained by dividing 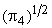 by by 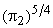 .
Let this parameter be designated by .
Let this parameter be designated by 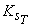 as as
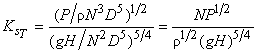 |
(3.3) |
Similarly, a parameter involving N , Q and H but not D is obtained by divining 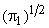 by by 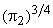 and is represented by and is represented by 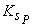 as as
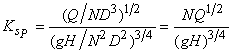 |
(3.4) |
Since the dimensionless parameters 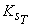 and and 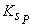 are
found as a combination of basic π terms, they must
remain same for complete similarity of flow in machines
of a homologous series. Therefore, a particular value
of are
found as a combination of basic π terms, they must
remain same for complete similarity of flow in machines
of a homologous series. Therefore, a particular value
of 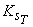 or or 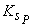 relates all the combinations of N , P and H or N , Q and H for which the flow conditions are similar in the machines of that homologous series. Interest naturally centers on the conditions for which the efficiency is a maximum. For turbines, the values of N , P and H , and for pumps and compressors, the values of N , Q and H are usually quoted for which the machines run at maximum efficiency. relates all the combinations of N , P and H or N , Q and H for which the flow conditions are similar in the machines of that homologous series. Interest naturally centers on the conditions for which the efficiency is a maximum. For turbines, the values of N , P and H , and for pumps and compressors, the values of N , Q and H are usually quoted for which the machines run at maximum efficiency.
The machines of particular homologous series, that is, of a particular shape, correspond to a particular value of 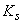 for their maximum efficient operation. Machines of different shapes have, in general, different values of for their maximum efficient operation. Machines of different shapes have, in general, different values of 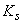 . Thus the parameter . Thus the parameter 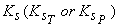 is referred to as the shape factor of the machines. Considering the fluids used by the machines to be incompressible, (for hydraulic turbines and pumps), and since the acceleration due to gravity dose not vary under this situation, the terms g and is referred to as the shape factor of the machines. Considering the fluids used by the machines to be incompressible, (for hydraulic turbines and pumps), and since the acceleration due to gravity dose not vary under this situation, the terms g and 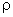 are taken out from the expressions of are taken out from the expressions of 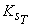 and and 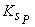 . The portions left as . The portions left as 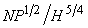 and and 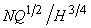 are termed, for the practical purposes, as the specific speed are termed, for the practical purposes, as the specific speed 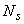 for turbines or pumps. Therefore, we can write, for turbines or pumps. Therefore, we can write,
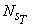 (specific speed for turbines) = (specific speed for turbines) =
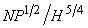 |
(3.5) |
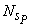 (specific speed for turbines) = (specific speed for turbines) =
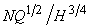 |
(3.6) |
The name specific speed for these expressions has a little justification. However a meaning can be attributed from the concept of a hypothetical machine. For a turbine, 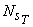 is the speed of a member of the same homologous series as the actual turbine, so reduced in size as to generate unit power under a unit head of the fluid. Similarly, for a pump, is the speed of a member of the same homologous series as the actual turbine, so reduced in size as to generate unit power under a unit head of the fluid. Similarly, for a pump, 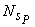 is speed of a hypothetical pump with reduced size but representing a homologous series so that it delivers unit flow rate at a unit head. The specific speed is speed of a hypothetical pump with reduced size but representing a homologous series so that it delivers unit flow rate at a unit head. The specific speed  is, therefore, not a dimensionless quantity. is, therefore, not a dimensionless quantity.
The dimension of 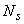 can
be found from their expressions given by Eqs. (3.5)
and (3.6). The dimensional formula and the unit
of specific speed are given as follows: can
be found from their expressions given by Eqs. (3.5)
and (3.6). The dimensional formula and the unit
of specific speed are given as follows:
| Specific speed |
Dimensional formula |
|
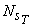 (turbine) (turbine) |
|
kg 1/2/ s5/2 m1/4 |
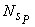 (pump) (pump) |
|
|
The dimensionless parameter 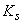 is often known as the dimensionless specific speed to distinguish it from is often known as the dimensionless specific speed to distinguish it from 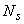 . .
|