A state x of a system is said to be controllable if the state can be reached from any initial state x₀ within a finite time interval and by using a finite control-effort. It can be proved that the controllability of a n -th order plant is possible when the controllability matrix given below is of full-rank n . The controllability matrix is defined as

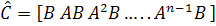(25.1)

It is easier to obtain the controllability matrix and design a full-state feedback controller when the state-space representation of the system is in the following form (known as controller canonical form).

controller canonical form

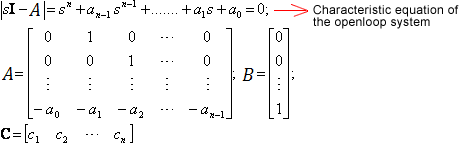(25.2)