THREE DIMENSIONAL RECONSTRUCTION FROM SCHLIEREN DATA
The schlieren image yields gradients of refractive index and hence concentration. As discussed in Section Data Reduction, the gradient information can be integrated to yield data in terms of concentration itself. Subsequently, the concentration field on selected horizontal planes above the growing crystal can be reconstructed using principles of tomography.
Here, the left side of Equation 3 (Lecture 26) is interpreted as projection data of the refractive index gradient.
The schlieren images have been utilized to reconstruct the concentration field on horizontal planes above the crystal. For this purpose, images have been recorded at four different view angles. Since these images are time-separated, the present study is restricted to a steady convection conditions. Minor changes in the concentration field with time have been accounted for by averaging a sequence of four successive images. The schlieren images recorded during the initial transients as well as late stages of growth when convection is unsteady have not been utilized for three dimensional reconstruction.
Computed Tomography
Tomography has been used in the present work to reconstruct two-dimensional concentration field over individual horizontal planes from their one-dimensional projections. The third dimension is filled by moving from one plane to the next in the third (vertical) direction. The recording configuration shown in Figure 5.16 is the parallel beam geometry. The convolution back projection (CBP) algorithm has been used in the present study for tomographic reconstruction. Significant advantages of this method include its non-iterative character, availability of analytical results on convergence of the solution with respect to the projection data, and established error estimates.

Figure 5.16: Schematic drawing of data collection using parallel beam geometry. S- source, D-Detector, s-perpendicular distance from the center of the object to the ray, 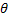 -view angle, and -view angle, and 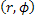 - polar coordinates. - polar coordinates.
|