| |
Data reduction
The present section examines the suitability of the three optical techniques of the present study for quantitative analysis of the convective field within a rectangular cavity and during crystal growth. An aspect shared by the three techniques is that they generate projection data, namely information that is integrated in the direction of propagation of the light beam. The result of analysis is thus a concentration (or temperature) that is ray-averaged over the length 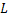 of the growth chamber. The steps involved in the analysis of the experimental images are briefly discussed below. of the growth chamber. The steps involved in the analysis of the experimental images are briefly discussed below.
Refractive index techniques depend on the fact that for a transparent material, refractive index and density share a unique relationship, called the Lorenz-Lorentz formula. In addition, if pressure changes within the fluid region are small, density will be uniquely related to solute concentration and temperature. The functionality is often linear since changes in concentration and temperature are often small in many applications. In rectangular cavity experiments, temperature is the only dependent variable and density (and hence refractive index) relates to temperature. Though crystal growth is driven by the cooling process of the solution, the ramp rate is often small enough for thermal equilibrium to prevail for short periods of time throughout the experiment. In the present analysis, it is assumed that the aqueous solution has negligible temperature gradients. Hence, refractive index becomes a measure of concentration itself. The material property that determines the sensitivity of the optical measurement is 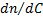 where where 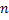 is the refractive index and is the refractive index and  is solute concentration. is solute concentration.
|