The second order moment of 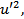 namely the mean square. The third moment namely the mean square. The third moment 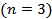 is called the skewness factor. This is called the skewness factor. This 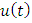 is zero for a Gaussian signal. The fourth moment is zero for a Gaussian signal. The fourth moment 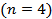 is called flatness factor or Kurtosis. Note that as is called flatness factor or Kurtosis. Note that as 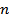 increases the accuracy with which increases the accuracy with which 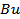 is determined for large is determined for large 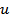 becomes critical. Typical examples where the skewness and flatness factors are respectively large as shown in Figure 1.13. becomes critical. Typical examples where the skewness and flatness factors are respectively large as shown in Figure 1.13.
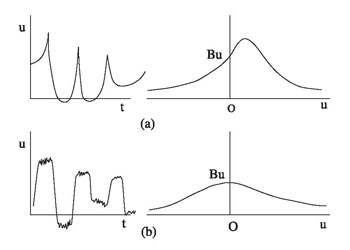
Figure 1.13: Signals with Large Skewness (a) and Large Flatness (b). |
The cross correlation 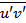 is determined in terms of PDF as is determined in terms of PDF as
where 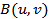 is called the joint probability density function. It is defined as the fraction of the time for which is called the joint probability density function. It is defined as the fraction of the time for which 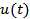 lies between lies between 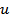 and and 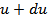 and and 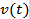 between between 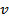 and and 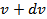 simultaneously. Autocorrelation can be determined in terms of simultaneously. Autocorrelation can be determined in terms of 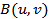 by identifying by identifying 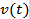 as as 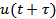 . .
Note that the PDF approach evaluates integrals in the amplitude domain alone. In comparison, the autocorrelation function represents time-domain statistics; the spectra are descriptors of the flow field in the frequency domain.
|