Window Correction
For visualization of the concentration field by the schlieren technique, circular optical windows have been fixed on the walls of the growth chamber at opposite ends. THe optical window employed in the present discussion of crystal growth is of finite thickness (5 mm) and the index of refraction of its material (BK-7) is considerably different from that of a KDP solution and air. The light beam emerging out of the KDP solution with an angular deflection 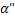 due to the variable concentration gradients in the growth chamber again undergoes refraction before finally emerging into the surrounding environment. The contribution of refraction of light at the confining optical windows can be accounted for by applying a correction factor in Equation 11 as discussed below. due to the variable concentration gradients in the growth chamber again undergoes refraction before finally emerging into the surrounding environment. The contribution of refraction of light at the confining optical windows can be accounted for by applying a correction factor in Equation 11 as discussed below.
The laser beam strikes the second optical window fixed on the growth chamber at an angle after undergoing refraction due to variable concentration gradients in the vicinity of the growing KDP crystal. The optical windows are made of BK-7 material with index of refraction 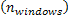 equal to 1.509. The refractive index of the KDP solution at an average temperature of equal to 1.509. The refractive index of the KDP solution at an average temperature of 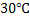 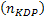 is equal to 1.355 and for air is equal to 1.355 and for air 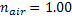 . Let . Let 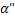 be the angular deflection of the beam purely due to the presence of concentration gradients in the vicinity of the growing crystal as shown schematically in Figure 5.6. be the angular deflection of the beam purely due to the presence of concentration gradients in the vicinity of the growing crystal as shown schematically in Figure 5.6.
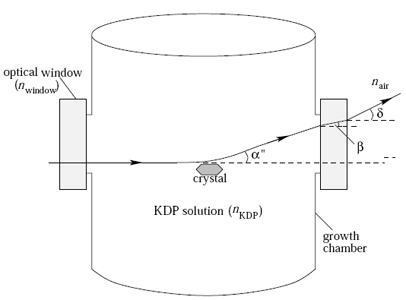
Figure 5.6: Schematic drawing showing the path of the light beam and the corresponding angles of deflection as it passes through the growth chamber. (Dimension in the figure are exaggerated for clarity).
The beam strikes the second optical window at this angle. Let 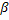 be the angle at which the laser beam emerges out of the second optical window. The angle at which the laser beam emerges out of the second optical window can be determined in terms of be the angle at which the laser beam emerges out of the second optical window. The angle at which the laser beam emerges out of the second optical window can be determined in terms of 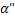 using Snell's law as using Snell's law as
|