The effective time constant of a pitot tube can be determined by considering together the effect of lumping density changes and spatial variations in velocity. From lumped analysis the time constant 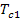 is is
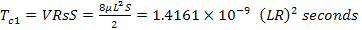
for air flow. Thus the value of time constant 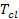 for a pitot tube obtained from lumped analysis is a function of both the radius and the tube length. for a pitot tube obtained from lumped analysis is a function of both the radius and the tube length.
Consider spatial variation next. The associated time constant is 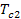 . The attenuation factor . The attenuation factor 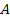 can be expressed as the reciprocal of the equivalent resistance, namely can be expressed as the reciprocal of the equivalent resistance, namely
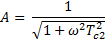
Values of 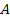 for each frequency for each frequency 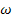 can then be obtained from Table 2 using this formula. The optimum value of can then be obtained from Table 2 using this formula. The optimum value of 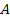 is determined using a least squares procedure through the reciprocal of the is determined using a least squares procedure through the reciprocal of the 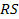 data in Table 2. This gives data in Table 2. This gives 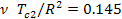 in dimensionless form and in dimensionless form and 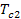 can be calculated for any value of can be calculated for any value of 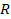 . . |