The radiation heat transfer process can be modeled (using the procedure of quasi-linearization) as
where 
with 
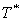denoting the guess value of 
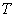calculated from the previous iteration or the specified guess. Therefore, the radiation integral becomes:
which can be handled like a convection term. Although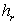 
varies over the length of the line element 
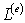due to variation in the value of 
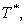it may not be worth the trouble if the element sizes are not too large. In such situations, one can set
where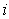 
and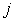 
are the nodes which make up the concerned boundary element.
A similar averaging procedure is often done for non-linear effects like temperature dependent properties, variable heat transfer coefficient at the boundary and variable heat flux at the boundary.
Congratulations, you have finished Lecture 22 To view the next lecture select it from the left hand side menu of the page or click the next button.
