Example 3: Buckling of columns (Euler-Bernoulli Equations)
The lateral displacement 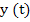 at position at position 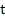 of a clamped-hinged bar of length of a clamped-hinged bar of length 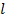 that is subjected to a load that is subjected to a load  may be approximated by the Euler-Bernoulli equations may be approximated by the Euler-Bernoulli equations
In the boundary conditions
The parameter 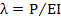 , where , where 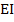 is the flexural rigidity of the bar. is the flexural rigidity of the bar.
This is an example of a Boundary Value Problem (BVP) for a fourth order linear ODE.
Note: Observe that 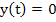 is a solution of this problem in all value of is a solution of this problem in all value of 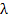 and and 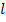 . A more interesting problem is to determine . A more interesting problem is to determine 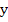 and those values of and those values of 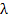 and and 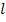 for which non-trivial for which non-trivial 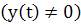 solutions exist. As such, this BVP is also a differential eigen value problem. Non- trivial solutions solutions exist. As such, this BVP is also a differential eigen value problem. Non- trivial solutions 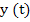 are called eigen functions and their corresponding values of are called eigen functions and their corresponding values of 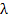 are eigen values. are eigen values.
|