Section III: Sequence Circuits for Synchronous Generator
The three-phase equivalent circuit of a synchronous generator is shown in Fig. 1.16. This is redrawn in Fig. 7.6 with the neutral point grounded through a reactor with impedance Zn . The neutral current is then given by
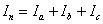
|
(7.32) |
Fig. 7.6 Equivalent circuit of a synchronous generator with grounded neutral.
The derivation of Section 1.3 assumes balanced operation which implies Ia + Ib + Ic = 0. As per (7.32) this assumption is not valid any more. Therefore with respect to this figure we can write for phase-a voltage as
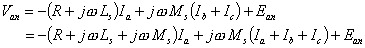
|
(7.33) |
Similar expressions can also be written for the other two phases. We therefore have
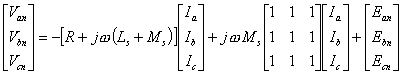
|
(7.34) |
Pre-multiplying both sides of (7.34) by the transformation matrix C we get
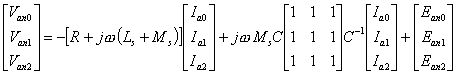
|
(7.35) |
Since the synchronous generator is operated to supply only balanced voltages we can assume that Ean0 = Ean2 = 0 and Ean1 = Ean . We can therefore modify (7.35) as
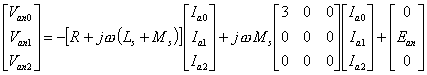
|
(7.36) |
We can separate the terms of (7.36) as
Furthermore we have seen for a Y-connected load that Va1 = V an1 , Va2 = Van2 since the neutral current does not affect these voltages. However Va0 = Van0 + Vn . Also we know that Vn = - 3ZnIa0 . We can therefore rewrite (7.37) as
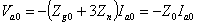
|
(7.40) |
The sequence diagrams for a synchronous generator are shown in Fig. 7.7.
Fig. 7.7 Sequence circuits of synchronous generator: (a) positive, (b) negative and (c) zero sequence.
|