Two dimensional stress at a point in a plane is defined by three components 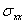 , , 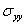 and and 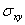 referred to orthogonal axes referred to orthogonal axes 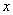 and and 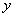 . The stress components represent the forces in the . The stress components represent the forces in the 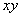 plane acting per unit area on the sides of an infinitesimal element of size plane acting per unit area on the sides of an infinitesimal element of size 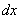 , , 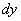 cut out on the slab. The tangential component cut out on the slab. The tangential component 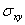 is same on both the sides is same on both the sides 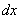 and and 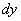 , because the torque of the resulting stresses on the element must be zero, this feature is known as the symmetric property as discussed in Lecture 2. , because the torque of the resulting stresses on the element must be zero, this feature is known as the symmetric property as discussed in Lecture 2.
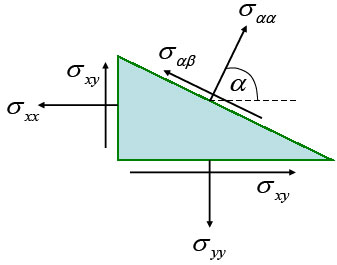
Figure 26.1
If we cut a small right-angled triangle OAB, then the normal and tangential tractions acting on the side AB are
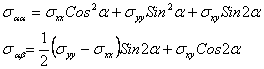 |
(26.1) |
The angle 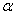 measures the inclination of the normal to AB with the x direction. The relation 26.1 generate the stress components w.r.t. axes 1 and 2 which are rotated by an angle measures the inclination of the normal to AB with the x direction. The relation 26.1 generate the stress components w.r.t. axes 1 and 2 which are rotated by an angle 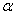 from the original directions xand y. from the original directions xand y. |