Moment of external forces:
Let's write the resultant moment about the OX axis of the body forces and the surface stresses acting on a surface S containing the volume V:
Using the relations for 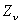 and and 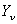 from 2.7, we have, from 2.7, we have,
Now, using of Green's Transformation, we obtain,
Gauss's Transformation
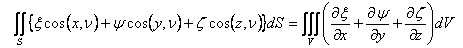
Proof of the Green's transformation can be found in Kreyszig
|
In order that the last integral(s) is(are) zero in any arbitrary region 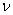 , we should have, , we should have,
Which implies that
the stress tensor 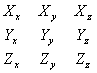 is symmetric. is symmetric. |