Longitudinal deformation of plates
Here we consider longitudinal deformations of plate subjected to either forces applied at the edges or by body forces applied on its plane. These form a special class of problems in which the plate does not undergo bending. Since the plate is sufficiently thin the deformations may be regarded as uniform over its thickness, the strain is then only a function of the 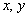 coordinates. The boundary conditions on both the surfaces of the plate are coordinates. The boundary conditions on both the surfaces of the plate are 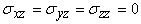 . In fact these conditions should hold even when surface forces are applied on the plate, because these forces are still smaller than the internal longitudinal stresses. Hence we have, . In fact these conditions should hold even when surface forces are applied on the plate, because these forces are still smaller than the internal longitudinal stresses. Hence we have,
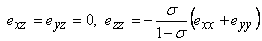 |
(17.1) |
Substituting these expressions into those of the non-zero components of the strain tensor, we have,
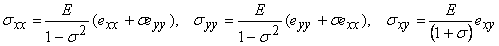 |
(17.2) |
If 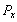 and and 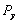 are the components of the external body forces applied per unit area of the plate, the general equations of equilibrium are, are the components of the external body forces applied per unit area of the plate, the general equations of equilibrium are,
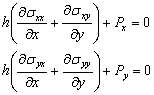 |
(17.3) |
which when combined with equation 10.17 to result in the following equilibrium relation in terms of the deformations in the plate.
 |
(17.4) |
Problem 3
Determine the deformation of a cylinder rotating uniformly about its axis.
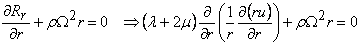 |
(17.5) |
Boundary condition: 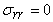 for for 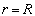
Ans: 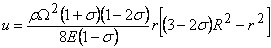
|
|