Concentric Cylinder Assemblage (CCA) Model
As we know, the unidirectional fibrous composite has fibres embedded in matrix material. The fibres are, in general, cylindrical in nature. Thus, Hashin and Rosen [1] introduced a micromechanical model in which the composite is represented as an assemblage of concentric cylinders. The inner cylinder represents the fiber and outer annulus is matrix. The fibres are considered to be infinitely long cylinders and matrix is considered to be continuous.
The model is shown in Figure 7.10(a). For each individual fibre of radius 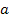 , there is associated an annulus of matrix material of inner radius , there is associated an annulus of matrix material of inner radius 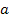 and outer radius and outer radius 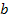 . The individual cylinder, thus formed, is called as a composite cylinder. This is shown in Figure 7.10 (b). It should be noted that the all cylinders do not have the same radii. The radii of each cylinder vary in a way such that they completely fill the composite volume. However, the ratio of the fiber cylinder to the outer radius of matrix cylinder is same for all cylinders. This leads to the fact that all composite cylinders have the same volume fractions. Further, the resulting material is transversely isotropic in nature. . The individual cylinder, thus formed, is called as a composite cylinder. This is shown in Figure 7.10 (b). It should be noted that the all cylinders do not have the same radii. The radii of each cylinder vary in a way such that they completely fill the composite volume. However, the ratio of the fiber cylinder to the outer radius of matrix cylinder is same for all cylinders. This leads to the fact that all composite cylinders have the same volume fractions. Further, the resulting material is transversely isotropic in nature.
The advantage of this model is that analysis of one composite cylinder is sufficient to determine the four out of five effective elastic moduli of a transversely isotropic material.
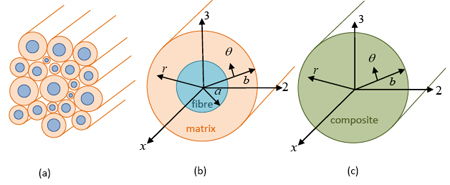 |
Figure 7.10: (a) Concentric cylinder assemblage model (b) composite cylinder and (c) homogeneous single cylinder |
|