1. Solve the London equation to determine the field variation inside a flat slab of very large surface area and of thickness d, placed in a magnetic field H parallel to the plane of the slab.

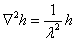
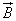
Start with . Write the solution as a combination of rising and falling exponentials with unknown coefficients. Impose the boundary condition that the tangential component of is continuous at the boundary. This determines the coefficients and the rest of the solution is given in the text.

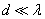
2. In the limit , why is complete flux expulsion not achieved ?

This is because the field enters in to the superconductor in a layer of thickness of the order of on the surface of the superconductor. If the size of the sample is small with respect to the penetration depth then a substantial portion of the sample has unexpelled flux.

3. What can you deduce about the current flow in a superconducting wire from the fact that B=0 within a superconductor ?

This implies that the current flows along the surface.

4. A long cylinder of a superconducting material is placed in an axial magnetic field. The critical field of this configuration is determined. Now, a sphere is made of the same material and it's behaviour in an applied magnetic field is measured. It is found that even at fields less than the critical field of the material, there are normal (non-superconducting) regions inside the sample? Explain this anomaly.

The magnetic field lines around a spherical sample are such that flux density is higher near the equator when the field is in the vertical direction. At the equator, the B-fieldis 1.5 times the applied H-field. Therefore when the applied field is 2/3 of the critical field, the B-field at the equator is equal to the critical field and regions near the equator start to become normal. On the other hand, for a long cylinder in an axial magnetic field, the demagnetisation field is zero and hence the B-field is uniform over the sample and equal to the applied field H.