1. A charge + Q is uniformly distributed over an annulus with an inner radius R and outer radius 2R. find the potential at a distance z from the annulus along its axis.

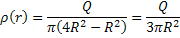
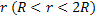
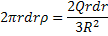
The charge density in the annulus is . Take a ring of radius  of width dr. The charge in the ring is . The potential due to this annulus at z is

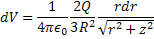
Integrating over the annulus, we have

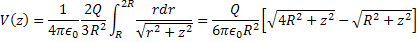
2. A positive charge +q is released from rest from the centre of the annulus of Problem 1 above. What is the final velocity attained by this mass.

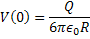
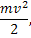
If a charge is released from the centre of the annulus (z=0) its potential energy is given by . Note that the potential energy at infinite distance is zero (take z>>R in the expression for V(z)). Thus at far distances, all the initial potential energy becomes the kinetic energy of the particle. Equating this to  the velocity can be found.

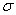
3. An infinite charged plane having a surface charge density is perpendicular to the z-axis at z=0. Obtain an expression for the electric potential and sketch the potential function.

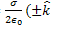
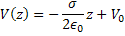
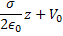
The electric field is given by ), where the upper sign is to be taken for  z>0 and the lower sign for z<0 (there is a discontinuity of the electric field at a charged surface). Thus above the plane, the potential is  and below the plane, it is ,, where  is an integration constant. Note that the potential is continuous at z=0, the boundary. A sketch of the potential is as follows :

 

 

 

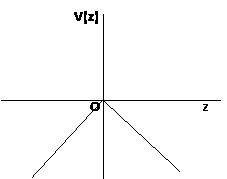
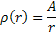
4. A sphere of radius R has a charge density   inside the sphere where r is the distance from the origin. Find the electric field and obtain an expression for the potential from the field.

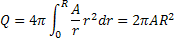
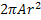
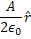
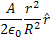
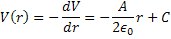
Total charge is obtained by integrating the charge density over the volume, . The amount of charge enclosed inside a spherical surface of radius r is . Using Gauss’s law, the electric field inside the sphere is  and outside the sphere it is ..  Because of spherical symmetry, the gradient is simply a differentiation with respect to r. 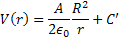Inside the sphere,   , where C is a constant  Outside the sphere, the potential is , with C’ being constant. 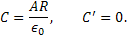The potential being continuous at the surface r=R, the constants must be chosen so that  Thus .
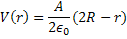