3.13.2 Mixed electronic ionic conduction in MgO
Here we demonstrate mixed ionic conduction in MgO. For working this out, we need to start with certain parameters. Assuming the temperature is 1600oC, for MgO, we can estimate
-
concentration of Schottky defect ≈ 1.4*1011cm3
-
concentration of electronic defects ≈ 3.6*109cm-3
-
Vacancies present are VMg'' and V0'' and VMg'' having higher mobility than V0'' .
Diffusion coefficient of magnesium vacancies is estimated as
At 1600oC, one can calculate the mobility from the Nernst-Einstein Equation i.e
However, at 1600oC
So, even though the vacancy concentration is larger than electron or hole concentration, conduction is dominated by electrons or holes simply because their mobility is orders of more than 6 order of magnitude higher.
However, in reality, MgO is prone to having impurities such as Al2O3 with Al concentrations of the order of 400 mole ppm or 2*1019cm-3. Using the defect reaction, one can write
Again we assume that the conduction is via Magnesium vacancies. Hence, Ionic conductivity is
However, electronic conductivity can be electron or hole dominated depending upon pO2 You can prove yourself that electrons dominate under reducing conditions while holes dominate under oxidizing conditions. For example, in normal atmosphere i.e. air (pO2 = 0.21atm), holes dominate. Hole concentration can be determined using the following defect reaction:
Here, from the mass conservation, we can see that nh = 2 VMg'' and hence the equilibrium constant is
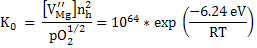
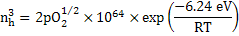
|
and hence
|
Using the above equation, nh can be determined at 1600oC air
So, the total conductivity is
Hence, we can now work out the ionic transference number as
-
The system shows mixed electronic and ionic conduction even when vacancy concentration is 105 times higher than electronic ( 2*1019cm-3 vs 5*1013cm-3).
-
p-type conduction can change to n-type as the pO2 decreases.
|