|
3.4 Mobility and Diffusivity
In addition to diffusivity, another useful term to describe conduction in ionic compounds and ceramics is mobility which is defined as velocity (v) of an entity per unit driving force (F) and is expressed as
The Force F can be defined as either of chemical potential gradient, electrical potential gradient, interfacial energy gradient, elastic energy gradient or any other similar parameter.
Absolute mobility
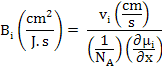 |
(3.18) |
Where
NA is Avogadro's Number,
μi is chemical potential in J/mol and x is the position in cm.
Chemical mobility
 |
(3.19) |
Electrical mobility
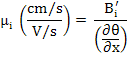 |
(3.20) |
For atomic transport, Einstein first pointed out that the most general driving force is the virtual force that acts on a diffusing atom or species and is due to negative gradient of the chemical potential or partial molar free energy. It is expressed as
Where μi is the chemical potential of i and NA is the Avogadro's Number.
Absolute mobility, Bi is given by
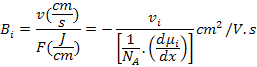 |
(3.22) |
To obtain the relation between mobility and diffusivity of species, i, we need to write the flux in a general form as a product of concentration, ci, and velocity, vi, i.e.
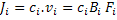 |
(3.23) |
Now, substituting for Fi from equation (3.21), we have
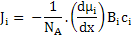 |
(3.24) |
Now, for an ideal solution with unit activity of species i , chemical potential can be expressed as
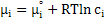 |
(3.25) |
where R is the gas constant. So, the change in the chemical potential can be written as
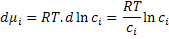 |
(3.26) |
OR
Substituting equation (3.27) into equation (3.24) leads to
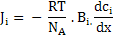 |
(3.28) |
If we compare the above equation with Fick's first law, which is equation (3.1), diffusivity of species i can be written as
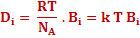 |
(3.29) |
The above equation is called Nernst Einstein Equation. |