Explanation GM=BM-BG
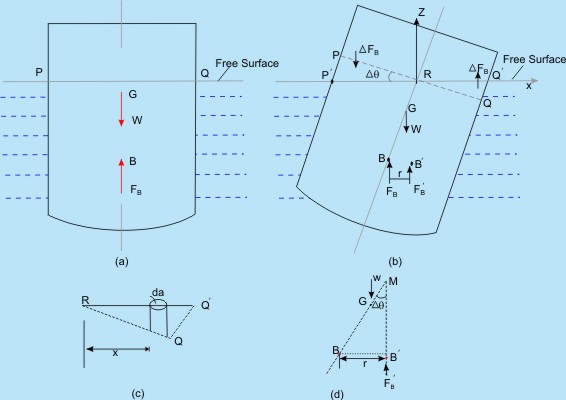
For equilibrium 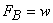
Due to symmetry of the situation, displaced volume remains unaltered and hence the buoyancy force
Thus, 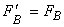
For couple calculation, 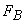 can be equivalently taken as a sum of can be equivalently taken as a sum of
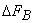 (upward) due to added volume of fluid RQQ' (upward) due to added volume of fluid RQQ' 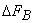 (downward) due to decreased volume RPP' (downward) due to decreased volume RPP'
Let DC be the couple due to these forces. Taking an element of area dA on the surface RQQ' at a distance x from the center line (as shown in fig (c) ). Corresponding volume element is x DθdA and the buoyant force is ρgxDθ dA. This produces the couple 2ρgx DθdA (due to symmetrically located element on RP' )
Therefore, Integrating 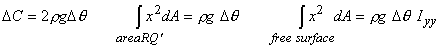
Now we have 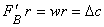 where r is moment arm of where r is moment arm of 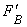 about B. about B.
Therefore, 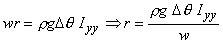
Now from fig (d) ; 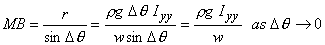
Thus 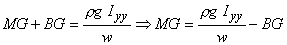
This is the metacentric height Example;
A hollow cylinder open at both ends has an internal diameter of 20cm, a wall thickness of 10 cm, and a length of 80cm. Given that the weight of the cylinder is 60kgf, comment on the stability of the cylinder.
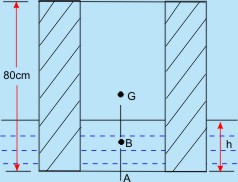
Let 'h' be the depth of submersion, then displaced volume will be
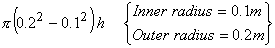
Assuming density of water to be 1000 kg/m3 , for equilibrium of the cylinder
Weight of the cylinder = weight of the displaced water
|
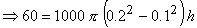
|
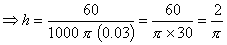
|
Therefore,
Therefore Metacentric Height, 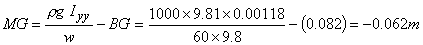 Therefore, MG<0 implies Cylinder is unstable
|