Hydrostatic Thrusts on Submerged Curved Surfaces
On a curved surface, the
direction of the normal changes from point to point, and hence
the pressure forces on individual elemental surfaces differ
in their directions. Therefore, a scalar summation of them
cannot be made. Instead, the resultant thrusts in certain
directions are to be determined and these forces may then
be combined vectorially. An arbitrary submerged curved surface
is shown in Fig. 5.3. A rectangular Cartesian coordinate system
is introduced whose xy plane coincides with the free surface
of the liquid and z-axis is directed downward below the x
- y plane.
Fig 5.3 Hydrostatic thrust on a Submerged Curved Surface
Consider an elemental area dA at a depth z from the surface of the liquid. The hydrostatic force on the elemental area dA is
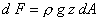 |
(5.11) |
and the force acts in a direction normal to the area dA. The components of the force dF in x, y and z directions are
Where l, m and n are the direction cosines of the normal to dA. The components of the surface element dA projected on yz, xz and xy planes are, respectively
Substituting Eqs (5.13a-5.13c) into (5.12) we can write
Therefore, the components of the total hydrostatic force along the coordinate axes are
where zc is the z coordinate of the centroid of area Ax and Ay (the projected areas of curved surface on yz and xz plane respectively). If zp and yp are taken to be the coordinates of the point of action of Fx on the projected area Ax on yz plane, , we can write
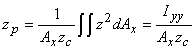
|
(5.16a) |
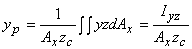 |
(5.16b) |
where Iyy is the moment of inertia of area Ax about y-axis and Iyz is the product of inertia of Ax with respect to axes y and z. In the similar fashion, zp' and x p' the coordinates of the point of action of the force Fy on area Ay, can be written as
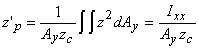
|
(5.17a) |
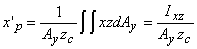 |
(5.17b) |
where Ixx is the moment of inertia of area Ay about x axis and Ixz is the product of inertia of Ay about the axes x and z.
We can conclude from Eqs (5.15), (5.16)
and (5.17) that for a curved surface, the component of hydrostatic
force in a horizontal direction is equal to the hydrostatic
force on the projected plane surface perpendicular to that
direction and acts through the centre of pressure of the projected
area. From Eq. (5.15c), the vertical component of the hydrostatic
force on the curved surface can be written as
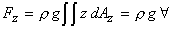 |
(5.18) |
where 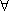 is the volume of the body of liquid within the region extending vertically above the submerged surface to the free surfgace of the liquid. Therefore, the vertical component of hydrostatic force on a submerged curved surface is equal to the weight of the liquid volume vertically above the solid surface of the liquid and acts through the center of gravity of the liquid in that volume. is the volume of the body of liquid within the region extending vertically above the submerged surface to the free surfgace of the liquid. Therefore, the vertical component of hydrostatic force on a submerged curved surface is equal to the weight of the liquid volume vertically above the solid surface of the liquid and acts through the center of gravity of the liquid in that volume.
|