Tanaka's Model
Tanaka's model is based on three state variables, which are strain (e), Temperature (T ) and Martensite volume fraction ( 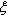 ). The relationship is given by ). The relationship is given by
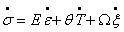 |
(34.1) |
where 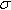 is the Piola-Kirchhoff stress, is the Piola-Kirchhoff stress,  is the Green strain, E is the Young's modulus, is the Green strain, E is the Young's modulus, 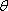 is the thermal expansion coefficient and is the thermal expansion coefficient and 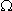 is the transformation modulus. The dot at the top denotes differentiation with respect to time. Further, the de-twinned Martensite volume fraction ( is the transformation modulus. The dot at the top denotes differentiation with respect to time. Further, the de-twinned Martensite volume fraction (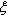 ) could be expressed as exponential function of stress and temperature as given below. ) could be expressed as exponential function of stress and temperature as given below.
For Austenite to Martensite transformation
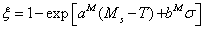 |
(34.2) |
where, 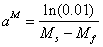 and and 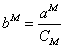
For Martensite to Austenite transformation
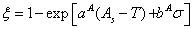 |
(34.3) |
where 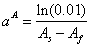 and and 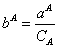 . .
The slopes CM and CA are indicated in Fig. 34.2.
Tanaka's model describes the behaviour of SMA qualitatively and is not suitable for engineering applications. Liang and Rogers improved this model to quantitatively describe the SMA behaviour. For avoiding the singularity appearing in the exponential form of Tanaka's Martensitic fraction model, they modified the expression by replacing the exponential with the cosine function. Brinson further improved Liang's model by introducing the fractions of stress-induced and temperature-induced Martensite as,
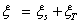 |
(34.4) |
where ξS is the fraction of stress-induced de-twinned Martensite with a single Martensite variant and ξT is the fraction of temperature-induced twinned Martensite with multiple variants. The cosine model is commonly referred as Liang-Brinson model and is given in the next slide.
|