While using a pitot tube for low speed measurement, two important sources of errors must be kept in mind: ( 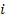 ) low Reynolds number error and ( ) low Reynolds number error and (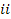 ) wall proximity error. At small values of Re, the fluid particles anticipate the presence of the probe wall and will have sufficient time to go around it. The curvature of the flow path and the divergence of the streamline accelerate the fluid locally and will reduce the static pressure below the free stream value. Hence ) wall proximity error. At small values of Re, the fluid particles anticipate the presence of the probe wall and will have sufficient time to go around it. The curvature of the flow path and the divergence of the streamline accelerate the fluid locally and will reduce the static pressure below the free stream value. Hence 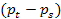 (measured) will be larger than the true value. Let (measured) will be larger than the true value. Let 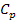 be the correction factor to the measured pressure difference. Typical values of be the correction factor to the measured pressure difference. Typical values of 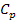 as a function of Re based on pitot tube diameter for a blunt-nosed pitot tube are given in Table 1 as a function of Re based on pitot tube diameter for a blunt-nosed pitot tube are given in Table 1
| Table 1: Correction Factor for a Pitot Probe at Low Reynolds Numbers |
Re |
5.000 |
10.000 |
20.000 |
40.00 |
60.00 |
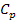
|
0.746 |
0.909 |
0.975 |
1.00 |
1.00 |
For 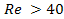 , , 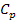 is nearly unity and no correction is required. For is nearly unity and no correction is required. For 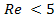 the correction factor is quite small and the uncertainty in its value limits the utility of the pitot tube. the correction factor is quite small and the uncertainty in its value limits the utility of the pitot tube.
The second source of error arises from the proximity of a wall. The distortion of the streamlines near the probe-wall region affects the local static pressure distribution as discussed earlier and it leads to the measurement of an incorrect velocity. Correction factors for this source of error are not readily available and accounting for the wall effect remains a challenge at this time.
|