Example 2.3

A diesel engine is operating at overall φ =0.6. Taking diesel fuel as  (CH₂)n and the combustion gas temperature and pressure as 2200 K and 60 atm calculate the initial oxidation rate of soot particle using Eq. 2.46 and the reaction constants as given for the NS-C mechanism. Estimate the period during which a soot particle of 50 nm dia would get completely oxidized.

Solution

Combustion gas conditions are given as, P = 60 atm, T = 2200 K Eq. 2.46 gives the soot oxidation rate as

 For 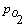, combustion stoichiometry for this engine may be written as

Total number of moles of products =1+1+1+9.4 = 12.4

Partial pressure of oxygen,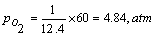

Reaction rate constants:

k_A  = 20 exp(-15,100/T) = 20 exp(-15100/2200) =  0.021 g/(cm2.s.atm)

k_C= 21.3 exp(2060/T) = 21.3 exp (2060/2200) = 54.3 atm-

The soot oxidation rate

Rate of particle burning in terms of rate of change of its radius,

Taking soot density ρ = 1.8 g/cm3

For a particle of dp = 50 nm diameter time duration required to burn completely,
 ∆t =  dp /2 (dr/dt)  = 50 x 10-9 x 100/ [2 x (2.56 x 10-3)] = 0.98 x 10-3 s or 0.98 ms  
At 2200 K and 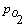= 4.84 bar, at 2000 rpm engine speed it would take about 12º CA to burn a 50 nm soot particle. Ans.