Vorticity-stream function approach
The vorticity-stream function method is one of the most popular methods for solving 2-D incompressible Navier-Strokes equations. The governing equations are
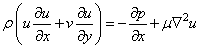 |
(24.1) |
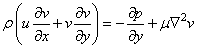 |
(24.2) |
and
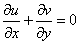 |
(24.3) |
As it was discussed earlier, it is difficult to deal with equation (24.1 and 24.2) due to the lack of presence of a separate equation for pressure 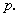 We introduce stream function We introduce stream function 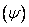 and vorticity and vorticity 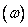 as as
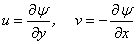 |
(24.4) |
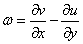 |
(24.5) |
We can readily see that existence of (24.4) automatically satisfies continuity equation (24.3) If we substitute the dependent variable with stream function, we shall not be concerned with equation (24.3) any more.
Invoking equation (24.4) into (24.5) we obtain Poisson equation.
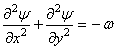 |
(24.6) |
Now we differentiate equation (24.1) with respect to  and equation (24.2) with respect to and equation (24.2) with respect to  . If we subtract differentiated equation (24.2) from differentiated equation (24.1) and rearrange the resulting equation, we shall obtain . If we subtract differentiated equation (24.2) from differentiated equation (24.1) and rearrange the resulting equation, we shall obtain
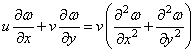 |
(24.7) |
This equation is the vorticity transport equation. Let us express the equation (24.6) in terms of finite difference quotient for 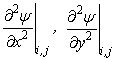 and and 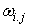 and putting and putting 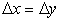
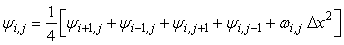 |
(24.8) |
|