Consider the probability matrix given below which denotes the communication network between 6 nodes marked 0, 1, 2, 3, 4 and 5.
|
0 |
1 |
2 |
3 |
4 |
5 |
0 |
1 |
0 |
0 |
0 |
0 |
0 |
1 |
½ |
0 |
½ |
0 |
0 |
0 |
2 |
0 |
½ |
0 |
½ |
0 |
0 |
3 |
0 |
0 |
½ |
0 |
½ |
0 |
4 |
0 |
0 |
0 |
½ |
0 |
½ |
5 |
0 |
0 |
0 |
0 |
0 |
1 |
A careful look at the matrix would make it apparent that following are the equivalence classes

Now if we have the matrix as
|
0 |
1 |
2 |
3 |
4 |
5 |
0 |
0 |
2 |
0 |
0 |
0 |
0 |
1 |
½ |
0 |
½ |
0 |
0 |
0 |
2 |
0 |
½ |
0 |
½ |
0 |
0 |
3 |
0 |
0 |
½ |
0 |
½ |
0 |
4 |
0 |
0 |
0 |
½ |
0 |
½ |
5 |
0 |
0 |
0 |
0 |
1 |
0 |
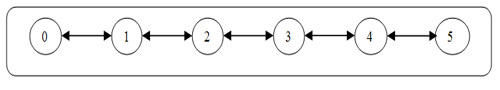
Then we have only one equivalent class and it is easily discernible that one can reach any state from any other state.
|