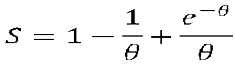Q10.1. (Source Levenspiel) Catalytic cracking of heavy oil to light fraction is a major operation in the petroleum industry. Silica-alumina catalyst is used. The catalyst deactivates rather rapidly due to coke formation. Therefore continuous regeneration is necessary to maintain satisfactory activity levels. The regeneration is brought about by burning off the coke. In this exercise a₁ and  a₂ refer to mean activities in the reactor and regenerator respectively.

Several commercial designs are available. Modern processes employ two fluidised beds. Solids are circulated between vessels (F_s). The circulation is achieved by a combination of pneumatic  and  gravity transfer.

The rate of solid circulation is the major design factor which limits the level of (a₂ – a₁) attainable. The cracking reaction is endothermic & the heat required to sustain the reaction is supplied by circulating solids since coke combustion is an exothermic reaction.

Most designs operate at (a₂ – a₁) at 0.6 to 0.85. Cracking reaction is conducted at 400°C & coke burning at 600°C using air. The maximum permissible coke level is generally 0.6 – 0.7 percent w/w of catalyst. In commercial practice the oxygen concentration at the exit from the regenerator is not different from that in the inlet. The volume change due to reaction is negligible.

The fluidized beds could be viewed as well mixed gas-solid contactor where gas & solids are  in  mixed flow in the reactor &  in the regenerator respectively.

The rate of activity generation in the two environments is given as

                      r₁ = - k₁ a   (reactor)    r₂ = k₂ (1 - a)  ( regenerator )

The mean catalyst activity may be defined as a = 1- C/C* where C is the mean loading of coke on catalyst in vessel & C* is the maximum possible loading in the vessel and a the mean activity

Q10.1.1. If (a₂- a₁) is 0.8 in a given system show that the optimum size ratio of the vessels is given as

                                              W₁/W₂  = ( k₂/k₁)** 0.5

Q10.1.2. It is desired to process 16 kg/s of feed in the reactor where the inlet gases have a concentration C_A0 = 5 g/l at 400°C.  The rate of formation of component A is

                               R_A = - k_r C_A  (g of A/g of cat. s)

Where  k_rC_A0 = 8.67 /s. Estimate the circulation of  solids F_s & solids holdup W₁ & W₂ for optimal choice of (W₁/W₂) if conversion of 60% is desired.

Q10.1.3 The size ratio W₁/W₂ is to be optimal. Estimate the values of a₁, a₂, t₁, t₂,  for the following values of rate constants
                   (Reactor)     k₁ = 0.05 /s at 400°C for C_A = 1.5 g/L

                        (Regenerator )  k₂= 0.04 /s at 500°C

Q10.2. The rate of generation of activity in the reactor & regenerator is given as,
       
                   r₁ = - k₁ am   (reactor)    r₂ = k₂ (1-a)ⁿ  ( regenerator )

Solve for m = 1 and n = 1. Herein ere activity is defined as the local values  a = 1 - C/C* where C is the coke on each particle & C*is the maximum permissible level on that local particle.

Q10.2.1. Determine the distribution of activity in the reactor & regenerator. Solve for m = 1 and n – 1.Then generalize for various values of m & n including  combinations  m > 1 & n > 1, m > 1 & n < 1 , m < 1 & n > 1 , m = 0 & n > 1, m = 0 & n < 1, m > 1 &  n = 0,  m < 1 & n = 0, m = 0 and n = 0.

Q10.2.2.  If m = 1 and n = 1 what is the mean activity of catalyst in the reactor & regeneration Express in terms of k₁t₁ = 1/α & k₂t₂  = 1/β. For optimal choice of α & C of 10.1.1, determine & and compare with results of 10.1.3.Note t₁  and t₂ are mean residence time in reactor and regenerator.

Q10.3. A fluidized bed reactor is to be designed for bringing about a reaction A(g) + B(s) = C(g) = D(s). Feed solids are of uniform size R and do not change in size due to reaction. The reaction follows the shrinking core model under reaction control. The reaction takes place in excess of A so that gas composition changes are not important. The reactor holds an inventory of solids of mass W₁. The reaction is essentially irreversible.

Q10.3.1. Set up the balance equation and show that the unreacted core radius in the particles leaving the reactor is distributing as below.
                                                

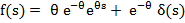
Where s = r/R, θ = 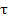/ t where  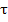is time for complete consumption of particle and t the mean solids residence time.  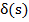refers to Dirac delta function at s = o .

Q10.3.2. Show that the mean S = r/R  and X_B can be given as
                                               

                                               

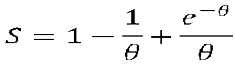
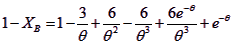
Q10.3.3. If time for complete consumption is 15 min and mean solids residence time is 30 min estimate mean solids conversion attainable.

Q10.4.Reactor-regenerator system
A catalytic reactor is used for continuous processing of gas A. the catalyst is a solid and undergoes rapid deactivation due to coking. In order to facilitate continuous processing the deactivating catalyst is continuously regenerated where coke is burnt off using air at high temperature. The regenerated catalyst is then returned to reactor.
Commercial practice engages two fluid beds wherein solids are assumed to be in mixed flow. Typically reactor is located at a higher level so that solids from reactor is transferred by gravity to regenerator. Then on regenerated catalyst is returned to reactor by pneumatic conveying.
The kinetics of deactivation in the reactor is given as r₁ = - k₁ a and regeneration kinetics r₂ = k₂ (1-a).

Q10.4.1. Set up the population balance model for the system and obtain the activity distributions.

Q10.4.2. If α (= 1/ k₁ t₁) = 2   and β = (1/k₂ t₂) = 3 find the activity distribution and mean activity in the reactor and regenerator where t₁ and t₂ are solid residence times in reactor and regenerator.

Q10.4.3. Overview the comparative advantages and disadvantages of fluidized bed and rotary kiln for gas-solid noncatalytic reactions.

Q10.5. Dual bed circulating reactor system
Carbonation of potassium carbonate with moist  carbon dioxide is carried out at 25 - 60  C and decomposition of potassium bicarbonate is carried out  at 100 – 200 C in a dual  fluidized bed circulating systems .
K₂CO₃ + H₂O + CO₂ = 2 KHCO₃                          (Reactor)
2 KHCO₃ =   K₂CO₃ + H₂O + CO₂                        (Regenerator)

Assuming that reactions take place throughout the particle the rate functions are
r₁ = - k₁ a        (in reactor) 
r₂ = k₂ (1-a)   (in regenerator)
where a refers to local carbonate. k₁ and k₂ depend on process conditions and in this case k₁ was found to be dependent on carbon dioxide concentration and k₂ on temperature only.

Q10.5.1. Set up the population balance for the system and determine the distribution of carbonate in the system.

Q10.5.2. Data has been gathered in laboratory fluidized bed over temperature range of 25-60 °C in reactor and 100-200 °C  in regenerator.

Experimental data (P.A.Joshi, PhD Thesis IIT Bombay 1986 ) is given below. α = 1.86 and β = 1.03 , a₁(mean)  = 0.451 and a₂ (mean)  = 0.873. Verify if the model developed can explain the data obtained.

Q10.5.3. Overview carbon dioxide recovery technology and comment on the suitability of the above method.

Q10.6. In forest environment organisms are born at a rate  B=  β 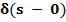 where β is constsnt. Organisms disappear either due to death or predation at a rate  D =  α S where  α  is constant.Here S refers to age of organisms.

Q10.6.1.Set up the equations and find the age distribution in the forest

Q10.6.2.Find the relationship between α and β that ensure steady state in the forest. Explain the physical significance .