The displacement continuity at 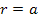 gives the relations gives the relations
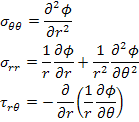 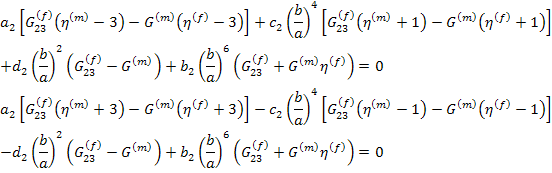 |
(7.262) |
The displacement continuity at 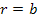 gives gives
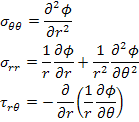 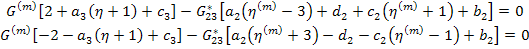 |
(7.263) |
The stress continuity at 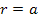 gives the following relations gives the following relations
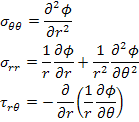 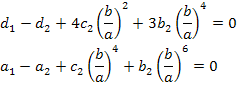 |
(7.264) |
And the stress continuity at 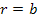 leads to leads to
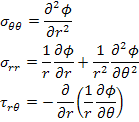 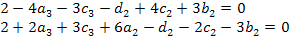 |
(7.265) |
Now the effective properties are determined from the equivalence of strain energy in heterogeneous media and in the equivalent homogeneous media. Thus, we write this condition as
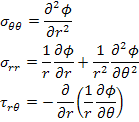 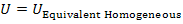 |
(7.266) |
The strain energies in the above equation can be given in terms of Eshelby formula.
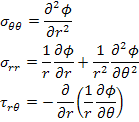 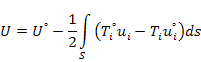 |
(7.267) |
where 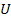 is the strain energy of a homogeneous medium containing an inclusion under the conditions of applied displacements. is the strain energy of a homogeneous medium containing an inclusion under the conditions of applied displacements. 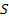 is the surface of the inclusion and is the surface of the inclusion and 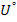 is the strain energy in the same medium when the medium does not contain any inclusion. Further, is the strain energy in the same medium when the medium does not contain any inclusion. Further, 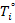 and and 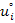 are the tractions and displacements on the surface when the medium has no inclusion. and are the tractions and displacements on the surface when the medium has inclusion. It should be noted that the context of three phase cylinder model the inclusion here it means the concentric cylinders. Thus, are the tractions and displacements on the surface when the medium has no inclusion. and are the tractions and displacements on the surface when the medium has inclusion. It should be noted that the context of three phase cylinder model the inclusion here it means the concentric cylinders. Thus, 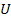 refers to the strain energy of the Figure 7.12(a). refers to the strain energy of the Figure 7.12(a). 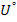 refers to the strain energy of the Figure 7.12(b) when the inclusion is replaced by the equivalent homogeneous material outside. However, this energy is refers to the strain energy of the Figure 7.12(b) when the inclusion is replaced by the equivalent homogeneous material outside. However, this energy is 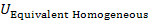 . .
Thus, we can write
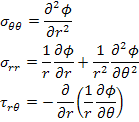  |
(7.268) |
Thus, from Equation (7.266) and Equation (7.268) we can write that
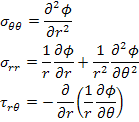 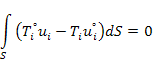 |
(7.269) |
|